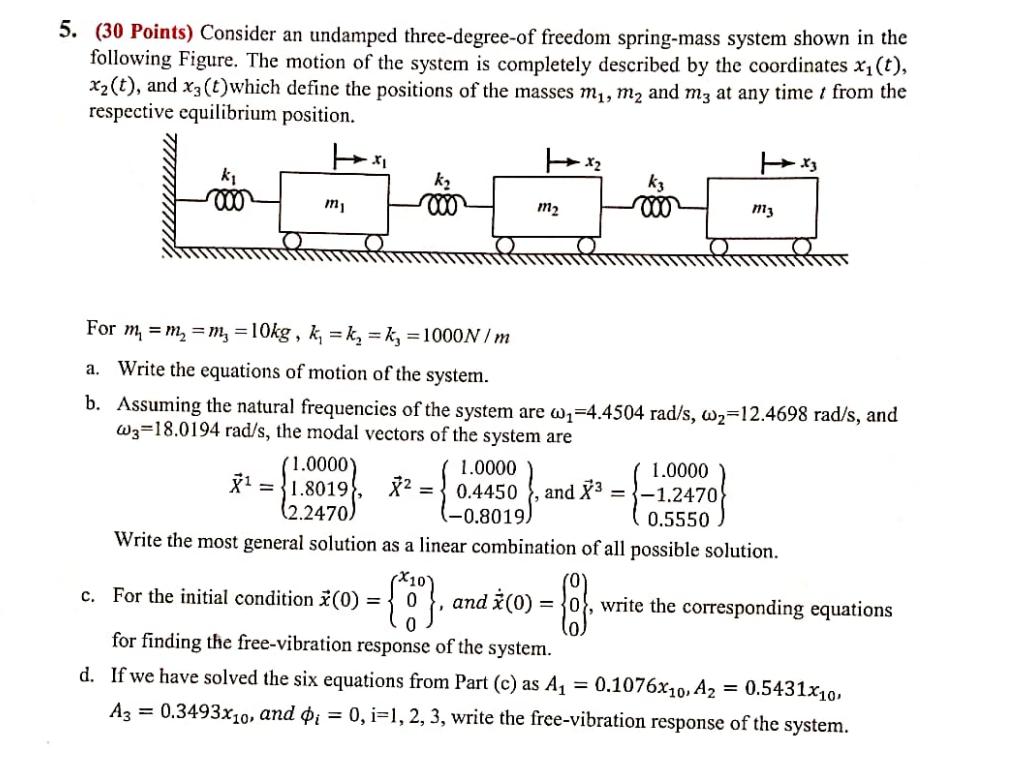
Consider an undamped three-degree-of freedom spring-mass system shown in the following Figure. The motion of the system is completely described by the coordinates x(t), x (t), and x3 (t)which define the positions of the masses m, m and m3 at any time from the respective equilibrium position. xH m coo c. For the initial condition (0) = For m m m = 10kg, k=k = k, = 1000N / m a. Write the equations of motion of the system. b. Assuming the natural frequencies of the system are w-4.4504 rad/s, w-12.4698 rad/s, and w3 18.0194 rad/s, the modal vectors of the system are X (1.0000) =1.8019 (2.2470) = m 1.0000 0.4450 -0.8019) 1.0000 and X3-1.2470 0.5550 Write the most general solution as a linear combination of all possible solution. X10 0 0 for finding the free-vibration response of the system. = 0.5431x10, d. If we have solved the six equations from Part (c) as A = 0.1076x10, A A3 = 0.3493x10, and p = 0, i=1, 2, 3, write the free-vibration response of the system. Ex H and * (0) M3 = - {8}. write the corresponding equations 26 = = 1.8019 A, Coswt+$, +0:4450 Azcosat +42-1:2470 Azcosust + 3 318.01947/340 x = 50 19390 4.4504t +0.2417 Cos12:46985-0-4356C0518-0194 x3 = 2.2470 A Coswit -0.8019A Cosust to: 555 Azcosist 23 = [0.24 18.605 24 18 c05 4.45046-04355 cos12.4698t +0.1.939 Casi p. 01947) 2010