Question
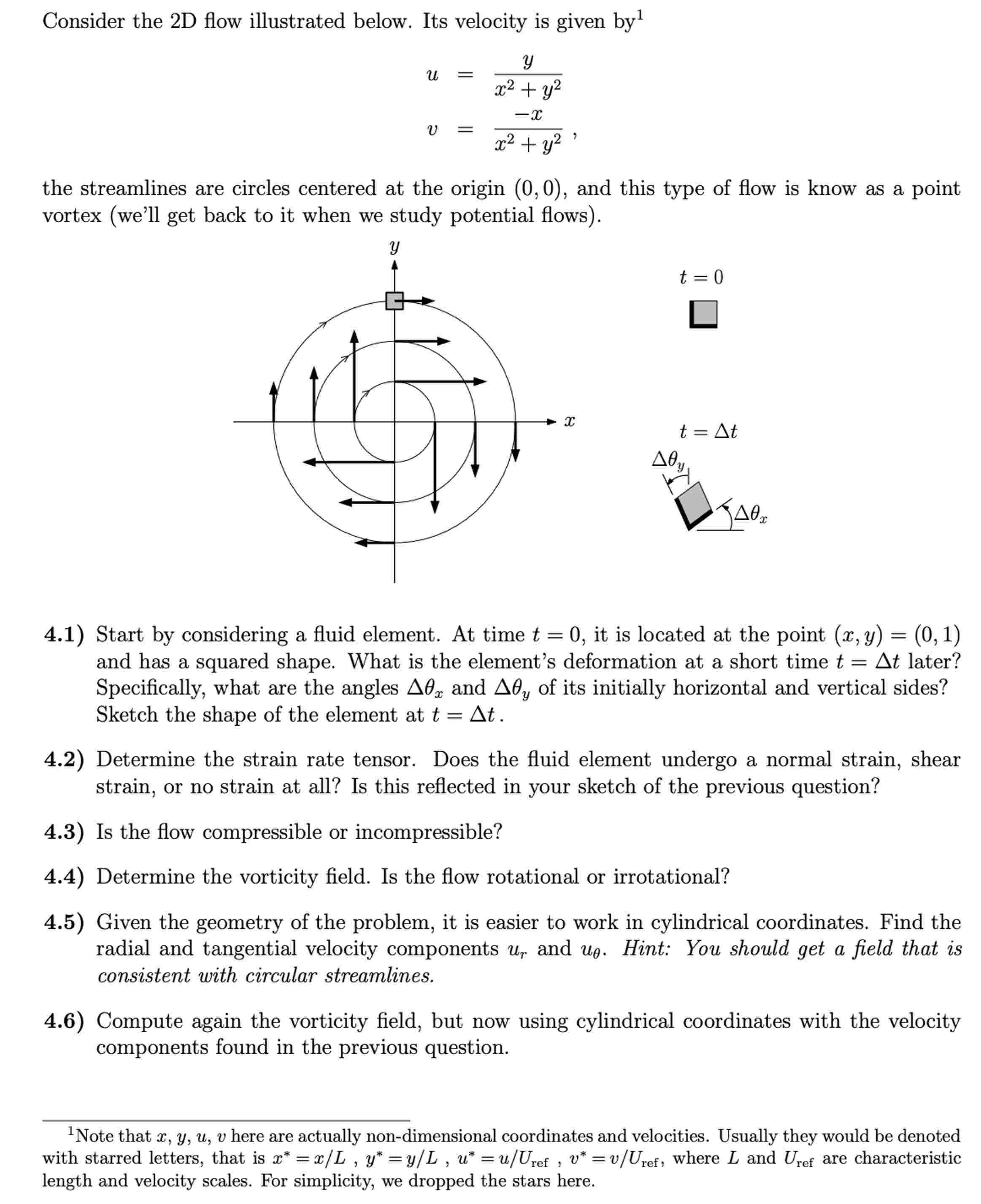
Consider the 2D flow illustrated below. Its velocity is given by ^(1) u=(y)/(x^(2)+y^(2)) v=(-x)/(x^(2)+y^(2)), the streamlines are circles centered at the origin (0,0)
Consider the
2Dflow illustrated below. Its velocity is given by
^(1)\
u=(y)/(x^(2)+y^(2))\ v=(-x)/(x^(2)+y^(2)),\ the streamlines are circles centered at the origin
(0,0), and this type of flow is know as a point\ vortex (we'll get back to it when we study potential flows).\
t=0\ \ 4.1) Start by considering a fluid element. At time
t=0, it is located at the point
(x,y)=(0,1)\ and has a squared shape. What is the element's deformation at a short time
t=\\\\Delta tlater?\ Specifically, what are the angles
\\\\Delta \\\\theta _(x)and
\\\\Delta \\\\theta _(y)of its initially horizontal and vertical sides?\ Sketch the shape of the element at
t=\\\\Delta t.\ 4.2) Determine the strain rate tensor. Does the fluid element undergo a normal strain, shear\ strain, or no strain at all? Is this reflected in your sketch of the previous question?\ 4.3) Is the flow compressible or incompressible?\ 4.4) Determine the vorticity field. Is the flow rotational or irrotational?\ 4.5) Given the geometry of the problem, it is easier to work in cylindrical coordinates. Find the\ radial and tangential velocity components
u_(r)and
u_(\\\\theta ). Hint: You should get a field that is\ consistent with circular streamlines.\ 4.6) Compute again the vorticity field, but now using cylindrical coordinates with the velocity\ components found in the previous question.\
^(1)Note that
x,y,u,vhere are actually non-dimensional coordinates and velocities. Usually they would be denoted\ with starred letters, that is
x^(**)=(x)/(L),y^(**)=(y)/(L),u^(**)=(u)/(U_(ref )),v^(**)=(v)/(U_(ref )), where
Land
U_(ref )are characteristic\ length and velocity scales. For simplicity, we dropped the stars here.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


