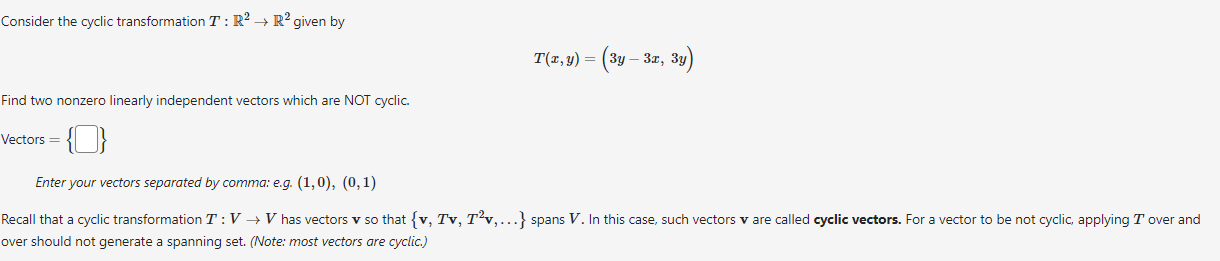
Consider the cyclic transformation T: R R given by T(x, y) = (3y 3x, 3y) Find two nonzero linearly independent vectors which are NOT cyclic. Vectors = Enter your vectors separated by comma: e.g. (1,0), (0,1) Recall that a cyclic transformation T: V V has vectors so that {v, Tv, T2v,...} spans V. In this case, such vectors v are called cyclic vectors. For a vector to be not cyclic, applying T over and over should not generate a spanning set. (Note: most vectors are cyclic.)