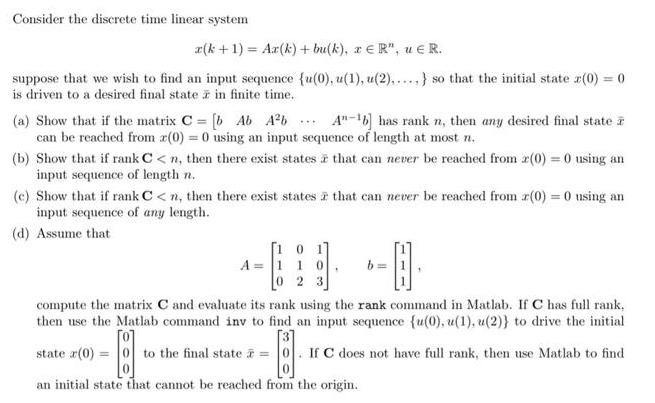
Consider the discrete time linear system x(k+1)= Ax(k) + bu(k), ze R", u R. H suppose that we wish to find an input sequence {u(0), u(1), u(2),...) so that the initial state z(0) = 0. is driven to a desired final state in finite time. (a) Show that if the matrix C = [b Ab Ab A-6] has rank n, then any desired final state can be reached from x(0) = 0 using an input sequence of length at most n. that can never be reached from 2(0) = 0 using an that can never be reached from r(0) = 0 using an *** (b) Show that if rank C