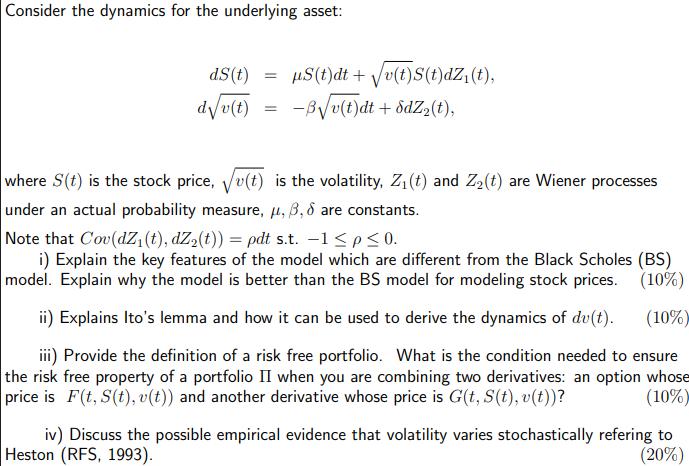
Consider the dynamics for the underlying asset: dS(t) = S(t)dt + v(t)S(t)dZ (t), dv(t) -Bv(t)dt + ddz(t), = where S(t) is the stock price, v(t) is the volatility, Z(t) and Z(t) are Wiener processes under an actual probability measure, , 3,6 are constants. Note that Cov(dZ (t), dZ(t)) = pdt s.t. -1 < p < 0. i) Explain the key features of the model which are different from the Black Scholes (BS) model. Explain why the model is better than the BS model for modeling stock prices. (10%) ii) Explains Ito's lemma and how it can be used to derive the dynamics of dv(t). (10%) iii) Provide the definition of a risk free portfolio. What is the condition needed to ensure the risk free property of a portfolio II when you are combining two derivatives: an option whose price is F(t, S(t), v(t)) and another derivative whose price is G(t, S(t), v(t))? (10%) iv) Discuss the possible empirical evidence that volatility varies stochastically refering to Heston (RFS, 1993). (20%)