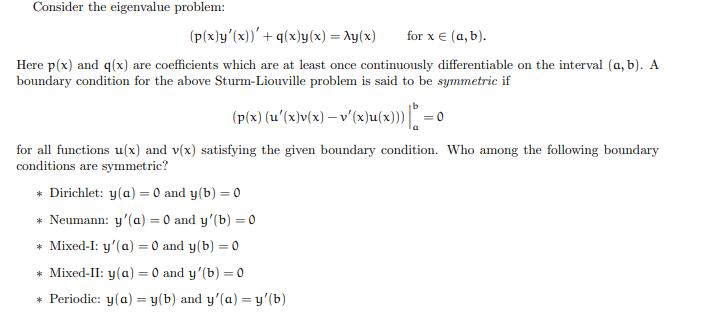
Consider the eigenvalue problem: (p(x)y'(x))' + q(x)y(x) = xy(x) for x (a, b). Here p(x) and q(x) are coefficients which are at least once continuously differentiable on the interval (a, b). A boundary condition for the above Sturm-Liouville problem is said to be symmetric if (p(x) (u'(x)v(x) v'(x)u(x))) = 0 for all functions u(x) and v(x) satisfying the given boundary condition. Who among the following boundary conditions are symmetric? * Dirichlet: y(a) = 0 and y(b) = 0 * Neumann: y'(a) = 0 and y'(b) = 0 * Mixed-I: y'(a) = 0 and y(b) = 0 * Mixed-II: y(a) = 0 and y'(b) = 0 * Periodic: y(a) = y(b) and y'(a)=y'(b)