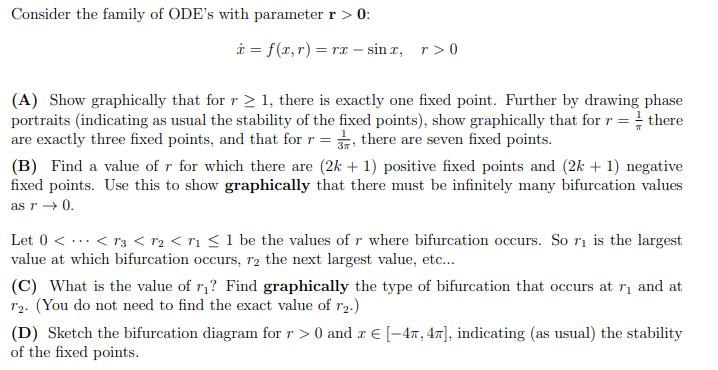
Consider the family of ODE's with parameter r > 0: if(x,r)=rx sina, r>0 - (A) Show graphically that for r 1, there is exactly one fixed point. Further by drawing phase portraits (indicating as usual the stability of the fixed points), show graphically that for r = there are exactly three fixed points, and that for r = 3, there are seven fixed points. (B) Find a value of r for which there are (2k + 1) positive fixed points and (2k + 1) negative fixed points. Use this to show graphically that there must be infinitely many bifurcation values as r0. Let 0 <