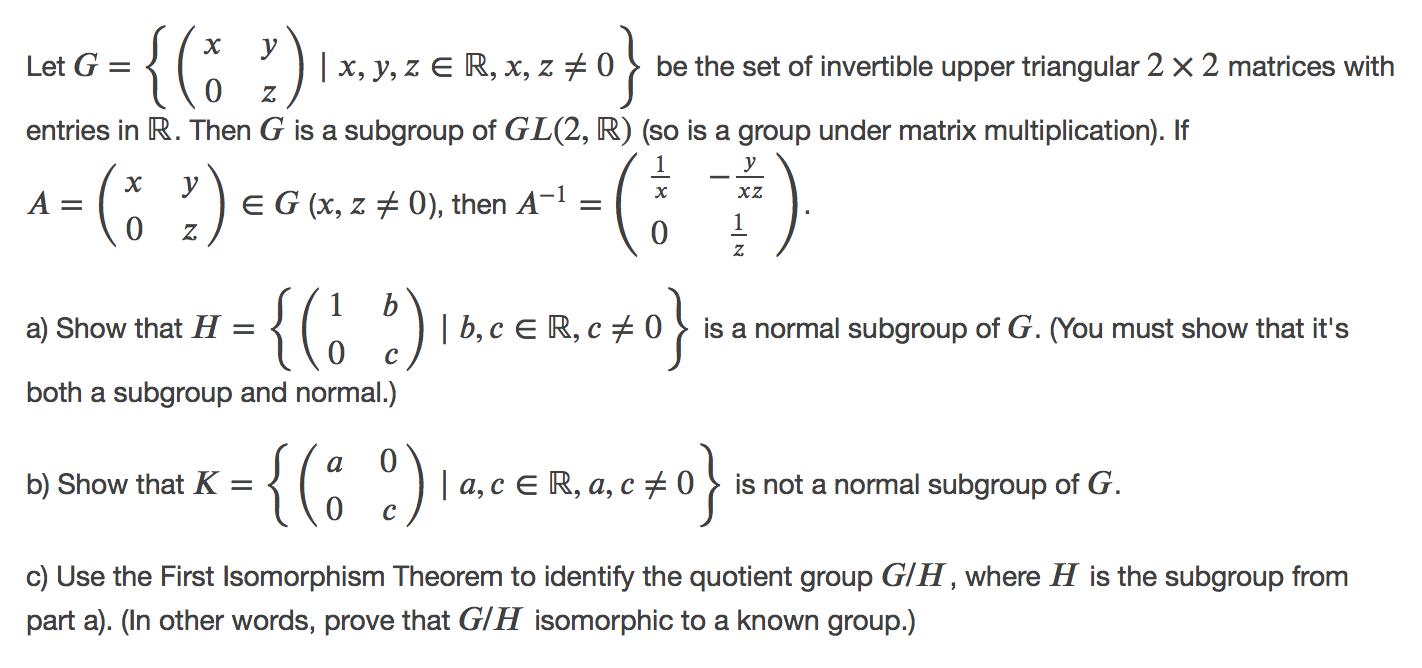
Let G = x | x, y, z ER, x, z 0 be the set of invertible upper triangular 2 2 matrices with {( 6 ) # 0 Z entries in R. Then G is a subgroup of GL(2, R) (so is a group under matrix multiplication). If y A = ( x y 0 Z. EG (x, z0), then A-1 = ( XZ Z a) Show that H = {(6 b) 1 b,c both a subgroup and normal.) b) Show that K = a 0 { ( ) | b, c R, c 0 is a normal subgroup of G. (You must show that it's |a, c ER, a, c0 + 0} is not a normal subgroup of G. c) Use the First Isomorphism Theorem to identify the quotient group G/H, where H is the subgroup from part a). (In other words, prove that G/H isomorphic to a known group.)