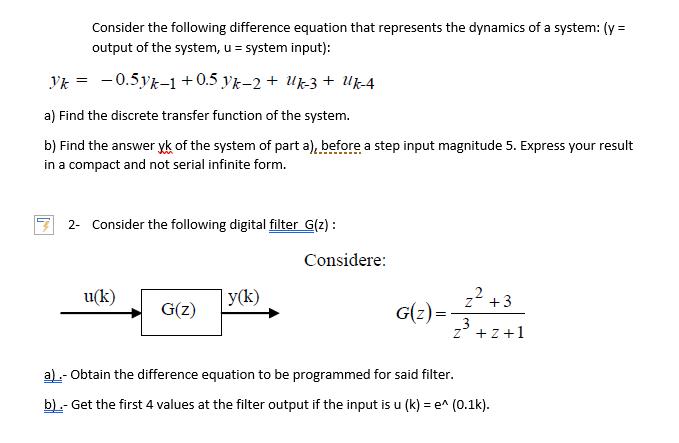
Consider the following difference equation that represents the dynamics of a system: (y = output of the system, u = system input): yk = -0.5yk-1 +0.5 yk-2 + Uk-3 + Uk-4 a) Find the discrete transfer function of the system. b) Find the answer yk of the system of part a), before a step input magnitude 5. Express your result in a compact and not serial infinite form. 2- Consider the following digital filter G(z) : u(k) G(z) y(k) Considere: G(3)=-3 +31 Z +2+1 a).- Obtain the difference equation to be programmed for said filter. b).- Get the first 4 values at the filter output if the input is u (k) = e^ ^ (0.1k).