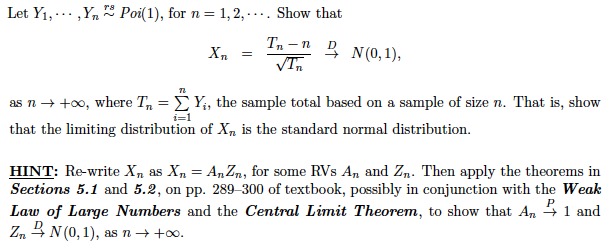
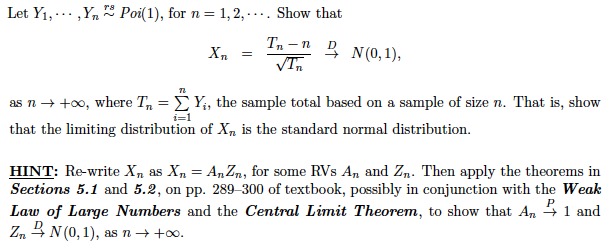
Question
Consider the following simplified scenario based on Who Wants to Be a Millionaire?, a game show in which the contestant answers multiple-choice questions that have

Consider the following simplified scenario based on Who Wants to Be a Millionaire?,
a game show in which the contestant answers multiple-choice questions that have 4
choices per question. The contestant (Fred) has answered 9 questions correctly already,
and is now being shown the 10th question. He has no idea what the right answers are
to the 10th or 11th questions are. He has one "lifeline" available, which he can apply
on any question, and which narrows the number of choices from 4 down to 2. Fred has
the following options available.
(a) Walk away with $16,000.
(b) Apply his lifeline to the 10th question, and then answer it. If he gets it wrong, he
will leave with $1,000. If he gets it right, he moves on to the 11th question. He then
leaves with $32,000 if he gets the 11th question wrong, and $64,000 if he gets the
11th question right.
(c) Same as the previous option, except not using his lifeline on the 10th question, and
instead applying it to the 11th question (if he gets the 10th question right).
Find the expected value of each of these options. Which option has the highest expected
value? Which option has the lowest variance?
10. Consider the St. Petersburg paradox (Example 4.3.13), except that you receive $n rather
than $2n if the game lasts for n rounds. What is the fair value of this game? What if
the payo? is $n2?
11. Martin has just heard about the following exciting gambling strategy: bet $1 that a
fair coin will land Heads. If it does, stop. If it lands Tails, double the bet for the next
toss, now betting $2 on Heads. If it does, stop. Otherwise, double the bet for the next
toss to $4. Continue in this way, doubling the bet each time and then stopping right
after winning a bet. Assume that each individual bet is fair, i.e., has an expected net
winnings of 0. The idea is that
1+2+22 + 23 + + 2n = 2n+1
The unit circle {(x, y) : x2 +y2 = 1} is divided into three arcs by choosing three random
points A, B, C on the circle (independently and uniformly), forming arcs between A and
B, between A and C, and between B and C. Let L be the length of the arc containing
the point (1, 0). What is E(L)? Study this by working through the following steps.
(a) Explain what is wrong with the following argument: "The total length of the arcs is
2?, the circumference of the circle. So by symmetry and linearity, each arc has length
2?/3 on average. Referring to the arc containing (1, 0) is just a way to specify one of
the arcs (it wouldn't matter if (1, 0) were re


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


