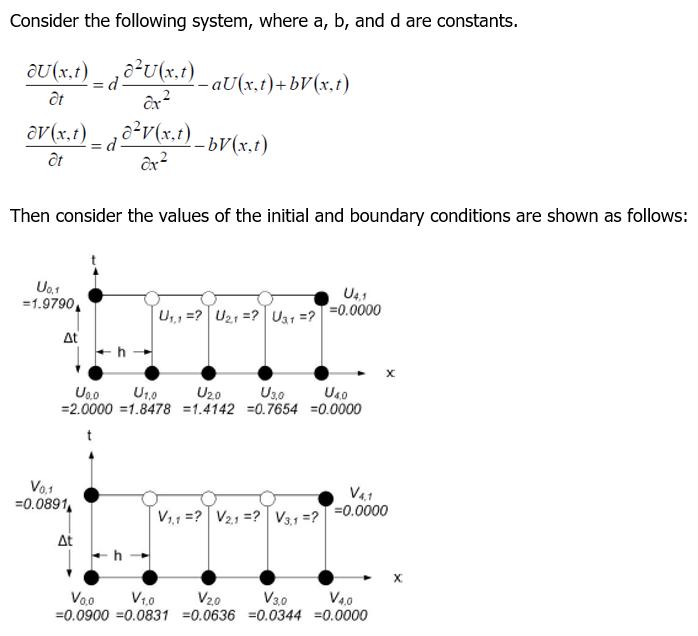
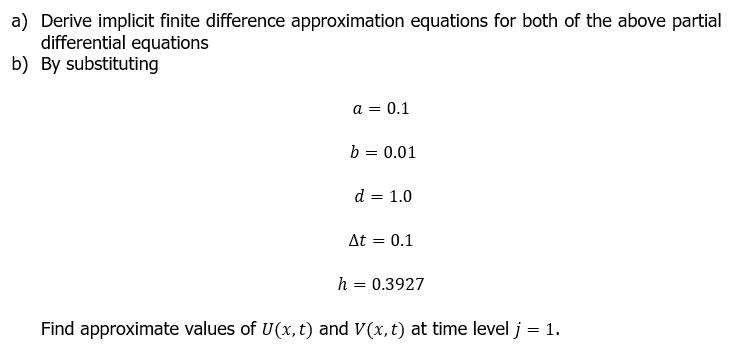
Consider the following system, where a, b, and d are constants. ) _dU(x,t) _ aU(x,t)+bV(x,t) ox JU(x,t) at av (x.t) _ qV(x,t) _ bv(x,t) at dx Then consider the values of the initial and boundary conditions are shown as follows: U0.1 =1.9790 At V0.1 =0.0891, U,1 ? U1 ? U1 =? U0.0 U1,0 U20 U3,0 U40 = 2.0000 = 1.8478 =1.4142 0.7654 =0.0000 t At U4,1 =0.0000 V.1 ? V21 ? V3,1 =? V4,1 =0.0000 V0,0 V1,0 V2,0 V3.0 V4.0 =0.0900 =0.0831 -0.0636 -0.0344 =0.0000 a) Derive implicit finite difference approximation equations for both of the above partial differential equations b) By substituting a = 0.1 b = 0.01 d = 1.0 At = 0.1 h = 0.3927 Find approximate values of U(x, t) and V(x, t) at time level j = 1.