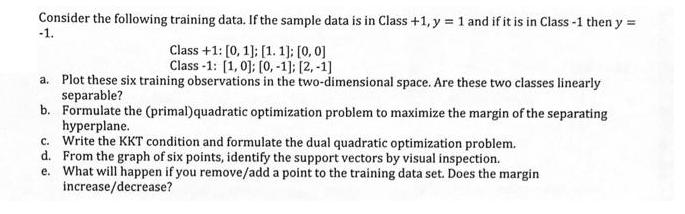
Consider the following training data. If the sample data is in Class +1, y = 1 and if it is in Class-1 then y = -1. Class +1: [0, 1]: [1. 1]; [0,0] Class-1: [1,0]; [0,-1]; [2,-1] a. Plot these six training observations in the two-dimensional space. Are these two classes linearly separable? b. Formulate the (primal) quadratic optimization problem to maximize the margin of the separating hyperplane. c. Write the KKT condition and formulate the dual quadratic optimization problem. d. From the graph of six points, identify the support vectors by visual inspection. e. What will happen if you remove/add a point to the training data set. Does the margin increase/decrease?