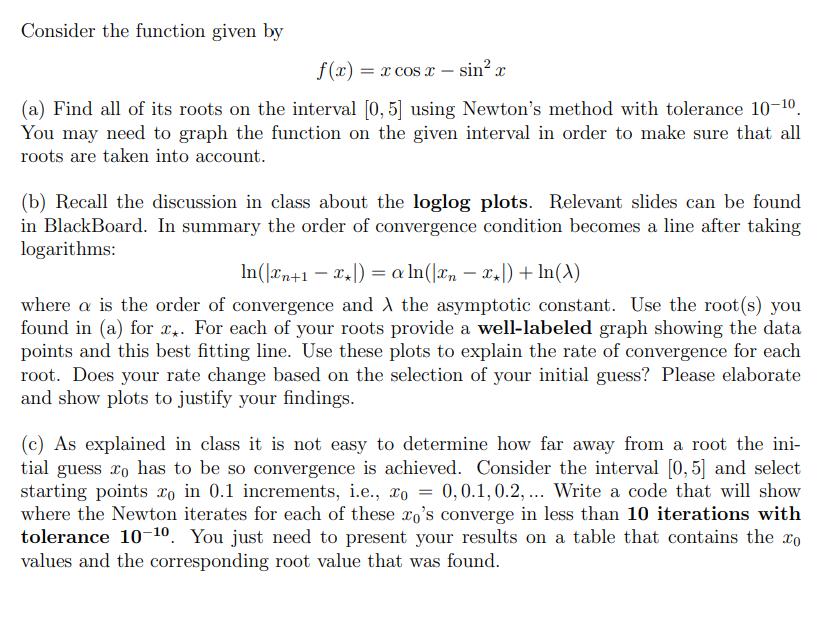
Consider the function given by f(x) = = x cos x - sin x (a) Find all of its roots on the interval [0,5] using Newton's method with tolerance 10-0. You may need to graph the function on the given interval in order to make sure that all roots are taken into account. (b) Recall the discussion in class about the loglog plots. Relevant slides can be found in BlackBoard. In summary the order of convergence condition becomes a line after taking logarithms: In(n+1)= a ln(xnx) + In(X) where a is the order of convergence and the asymptotic constant. Use the root(s) you found in (a) for x. For each of your roots provide a well-labeled graph showing the data points and this best fitting line. Use these plots to explain the rate of convergence for each root. Does your rate change based on the selection of your initial guess? Please elaborate and show plots to justify your findings. (c) As explained in class it is not easy to determine how far away from a root the ini- tial guess o has to be so convergence is achieved. Consider the interval [0,5] and select starting points ro in 0.1 increments, i.e., o = 0, 0.1, 0.2,... Write a code that will show where the Newton iterates for each of these ro's converge in less than 10 iterations with tolerance 10-10. You just need to present your results on a table that contains the co values and the corresponding root value that was found.