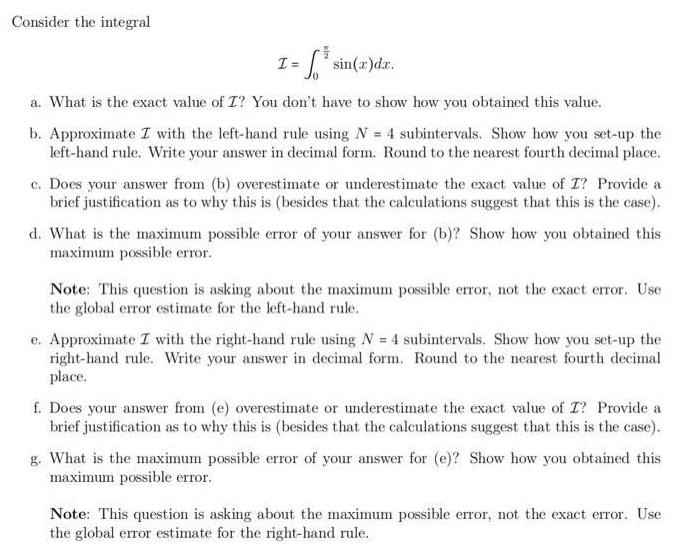
Consider the integral I= * sin(x)dx. a. What is the exact value of I? You don't have to show how you obtained this value. b. Approximate I with the left-hand rule using N = 4 subintervals. Show how you set-up the left-hand rule. Write your answer in decimal form. Round to the nearest fourth decimal place. c. Does your answer from (b) overestimate or underestimate the exact value of I? Provide a brief justification as to why this is (besides that the calculations suggest that this is the case). d. What is the maximum possible error of your answer for (b)? Show how you obtained this maximum possible error. Note: This question is asking about the maximum possible error, not the exact error. Use the global error estimate for the left-hand rule. e. Approximate I with the right-hand rule using N = 4 subintervals. Show how you set-up the right-hand rule. Write your answer in decimal form. Round to the nearest fourth decimal place. f. Does your answer from (e) overestimate or underestimate the exact value of I? Provide a brief justification as to why this is (besides that the calculations suggest that this is the case). g. What is the maximum possible error of your answer for (e)? Show how you obtained this maximum possible error. Note: This question is asking about the maximum possible error, not the exact error. Use the global error estimate for the right-hand rule.