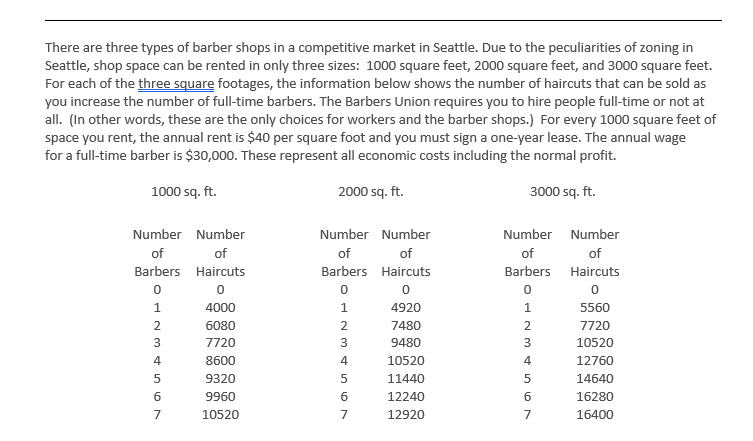
Consider the long run situation in the market where there is strong price competition, barber shops can change the square footage in their shop, and barber shops enter and exit the market. What do you anticipate will be the price in the long run? Based on that price, what will be the shop's optimal choice of shop size and number of barbers in the shop in the long run?Explain your logic.(4 points)

Net Price in Long Run equilibrium_______________.

Profit maximizing square footage in Long run equilibrium________________.

Profit maximizing number of workers in long run equilibrium _____________.

Explanation for your choices:

Answer:

Optimal workers for 1000 square feet is 2

Optimal workers for 2000 square feet is 3

Optimal workers for 3000 square feet is 5

Maximum profit for 1000 square feet is $9,440

Maximum profit for 2000 square feet is $640

Maximum profit for 3000 square feet is-$6,480 (minimum loss)

Explanation:

At profit maximization level, MC equals MR.

As long as the revenue from using another barber (MR) is greater than the cost of using that barber (MC), the shop will increase its profit by using more barbers to do more haircuts

Annual wage per barber = $30,000

Price per haircut = $18

Rent per square feet = $40

Size of shop = 1000, 2000, 3000 sq feet

Total Cost = (Rent per square feet * Size of shop) + (Annual wage per barber * Number of barbers)

Total Revenue = Number of Haircuts * Price per haircut

Marginal Cost = Total cost with n barbers - Total Cost with n-1 barbers

Marginal Revenue = Total Revenue with n barbers - Total Revenue with n-1 barbers

Size 1000 square feet

Updated the table below

Marginal Revenue with 2 barbers > Marginal Cost with 2 barbers

Marginal Revenue with 3 barbers

Optimal workers for 1000 square feet is 2

Maximum profit for 1000 square feet = Total Revenue with 2 barbers - Total Cost with 2 barbers

= $109,440 - $100,000 =$9,440

Maximum profit for 1000 square feet is $9,440

Size 2000 square feet

Updated the table below

Marginal Revenue with 3 barbers > Marginal Cost with 3 barbers

Marginal Revenue with 4 barbers

Optimal workers for 2000 square feet is 3

Maximum profit for 2000 square feet = Total Revenue with 3 barbers - Total Cost with 3 barbers

= $170,640 - $170,000 =$640

Maximum profit for 2000 square feet is $640

Size 3000 square feet

Updated the table below

Marginal Revenue with 5 barbers > Marginal Cost with 5 barbers

Marginal Revenue with 6 barbers

Optimal workers for 3000 square feet is 5

Maximum profit for 3000 square feet = Total Revenue with 5 barbers - Total Cost with 5 barbers

= $263,520 - $270,000 = -$6,480

Maximum profit for 3000 square feet is-$6,480 (minimum loss)

111ere are three types of barber shops in a competitive market in Seattle. Due to the peculia riti of zoning in Seattle, shop space can be rented in only three sizes: 1000 square feet, 2000 square feet, and 3000 square feet. For each of the three sgua re footages, the information below shows the number of haircuts that can be sold as you increase the number of fulltime barbers. The Barbers Union requira you to hire people fulltime or not at all. [In other words, these are the only choices for workers and the barber shops.] For every 1000 square feet of space you rent, the annual rent is $40 per square foot and you must sign a oneyear lease. The annual 1wage for a fulltime barber is $30,000. mere represent all economic costs including the normal prot. 1000 sq. ft. 2000 sq. ft. 3000 sq. ft. Number Number Number Number Number Number of of of of of of Barbers Haircuts Barbers Haircuts Barbers Haircuts 0 0 0 0 0 0 1 4cm 1 4920 1 5560 2 6030 2 2480 2 2220 3 2220 3 9480 3 10520 4 asap 4 10520 4 12260 5 9320 5 11440 5 14540 6 9960 E 12240 E 16280 2 10520 2 12920 2 164m