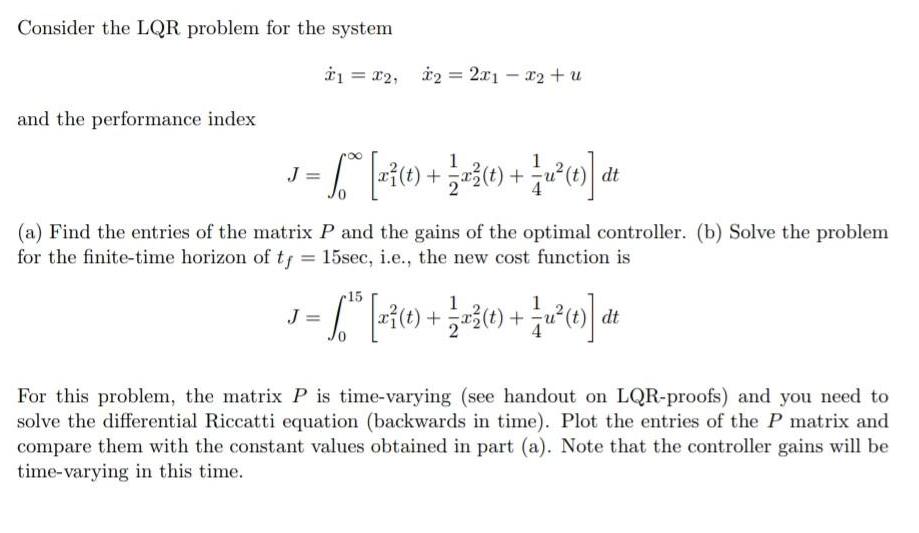
Consider the LQR problem for the system and the performance index *1 = x2, * = 2x - x + u J 1 = [ [ 2 (0) + 12 +7(0) + 1/201 (19)] 6 dt (a) Find the entries of the matrix P and the gains of the optimal controller. (b) Solve the problem for the finite-time horizon of tf = 15sec, i.e., the new cost function is J 115 [ 2300) + 1/ 130(0) + + 0 (19)] de dt For this problem, the matrix P is time-varying (see handout on LQR-proofs) and you need to solve the differential Riccatti equation (backwards in time). Plot the entries of the P matrix and compare them with the constant values obtained in part (a). Note that the controller gains will be time-varying in this time.