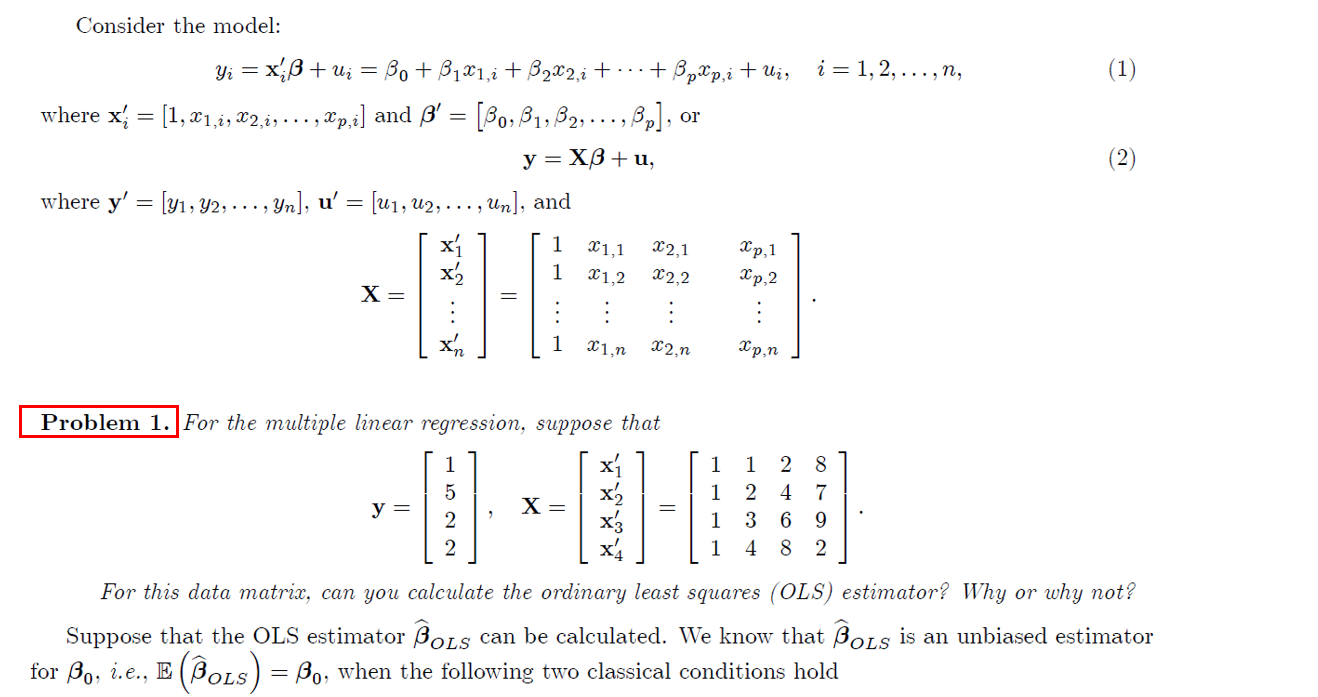
Consider the model: =1,2,...,n, (1) 2) Yi = x; 3+ u= Bo + B121,i + B2X2,i + ... + BpXp,i + Ui, where x; = [1, X1,1 , X2,1, . . . , Xp,i] and B' = [B0, B1, B2, ..., By], or y = XB+u, where y' = (y1, Y2, ..., yn], u' = [41, U2, ..., un], and x x'a Xp,2 X= 1 3p,1 1 1,1 21,2 X2,1 22,2 x, 21.n 22.n , Problem 1. For the multiple linear regression, suppose that 1 1 1 1 y = X= 28 4 7 6 9 8 2 x's 2 3 4 1 1 For this data matrix, can you calculate the ordinary least squares (OLS) estimator? Why or why not? Suppose that the OLS estimator Bols can be calculated. We know that Bols is an unbiased estimator for Bo, i.e., E (Bols) = Bo, when the following two classical conditions hold D1. {x1,1, X2,2, ..., Xp,i}=are nonstochastic, and D2. there exists a vector B = [B0,0,31,0, 32,0... , Bp,o] such that Yi = 30,0 + B1,081, i + 32,0X2,i +.... + Bp,0%p, i + Ein i=1,2,...,n, where E (@i) = 0, E (6) = o, and E (Eje;) = 0 for i, j = 1, 2, ..., n and i + j, Furthermore, under D1 and D2, Var (Bols) = Vots = of (X'X)"}, n 1 22 (yi i)? n- p - 1 i=1 is an unbiased estimator for om, and G (x'x)- BOLS (4) is an unbiased estimator for V BOLS' Problem 2. Consider the model: Yi = Bo + B1X1, + B2X2,i + Wig i = 1, 2, ...,n, Is Bols still an unbiased estimator for Bo when D1 and D2 hold in general but 1. E (6) = x, i, i.e., {ei} are not homoskedastic, but heteroskedastic? = 0 and B2,0 = 0, i.e., {21,1, X2,1}}=1 are not correlated with {yi}}=1? 3. there exists a vector B6 = [B0,0,1,0,B2,0] and + 0 such that 2. B1,0 70 Yi = Bo,o + B1,081, i + B2,0X2,i + YOX3,i + Ei, i=1,2,...,n, where {x3,i}}=1 are random variables such that E (23,i) = H3 + 0? Consider the model: =1,2,...,n, (1) 2) Yi = x; 3+ u= Bo + B121,i + B2X2,i + ... + BpXp,i + Ui, where x; = [1, X1,1 , X2,1, . . . , Xp,i] and B' = [B0, B1, B2, ..., By], or y = XB+u, where y' = (y1, Y2, ..., yn], u' = [41, U2, ..., un], and x x'a Xp,2 X= 1 3p,1 1 1,1 21,2 X2,1 22,2 x, 21.n 22.n , Problem 1. For the multiple linear regression, suppose that 1 1 1 1 y = X= 28 4 7 6 9 8 2 x's 2 3 4 1 1 For this data matrix, can you calculate the ordinary least squares (OLS) estimator? Why or why not? Suppose that the OLS estimator Bols can be calculated. We know that Bols is an unbiased estimator for Bo, i.e., E (Bols) = Bo, when the following two classical conditions hold D1. {x1,1, X2,2, ..., Xp,i}=are nonstochastic, and D2. there exists a vector B = [B0,0,31,0, 32,0... , Bp,o] such that Yi = 30,0 + B1,081, i + 32,0X2,i +.... + Bp,0%p, i + Ein i=1,2,...,n, where E (@i) = 0, E (6) = o, and E (Eje;) = 0 for i, j = 1, 2, ..., n and i + j, Furthermore, under D1 and D2, Var (Bols) = Vots = of (X'X)"}, n 1 22 (yi i)? n- p - 1 i=1 is an unbiased estimator for om, and G (x'x)- BOLS (4) is an unbiased estimator for V BOLS' Problem 2. Consider the model: Yi = Bo + B1X1, + B2X2,i + Wig i = 1, 2, ...,n, Is Bols still an unbiased estimator for Bo when D1 and D2 hold in general but 1. E (6) = x, i, i.e., {ei} are not homoskedastic, but heteroskedastic? = 0 and B2,0 = 0, i.e., {21,1, X2,1}}=1 are not correlated with {yi}}=1? 3. there exists a vector B6 = [B0,0,1,0,B2,0] and + 0 such that 2. B1,0 70 Yi = Bo,o + B1,081, i + B2,0X2,i + YOX3,i + Ei, i=1,2,...,n, where {x3,i}}=1 are random variables such that E (23,i) = H3 + 0