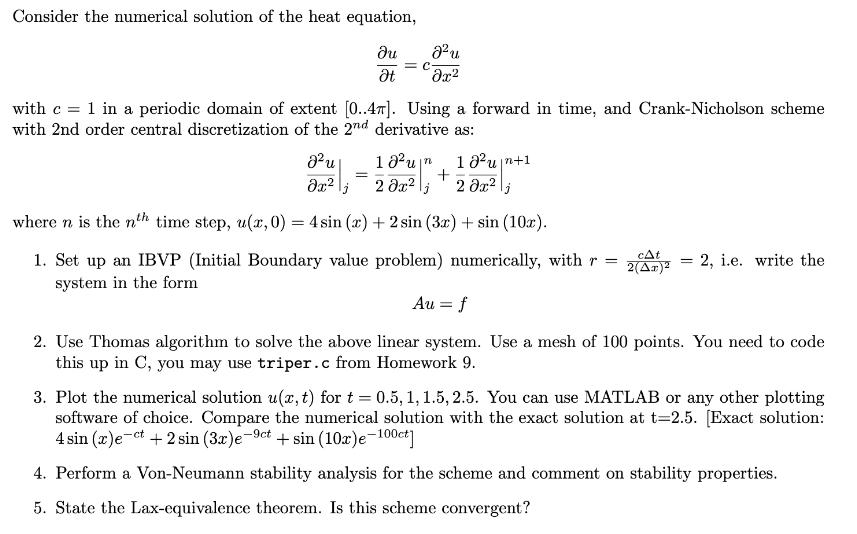
Consider the numerical solution of the heat equation, t = C 2 with c = 1 in a periodic domain of extent [0..4]. Using a forward in time, and Crank-Nicholson scheme with 2nd order central discretization of the 2nd derivative as: Ju 2 = 10un + 12n+1 2 x j 2 x j where n is the nth time step, u(x, 0) = 4 sin (x) + 2 sin (3x) + sin (10x). 1. Set up an IBVP (Initial Boundary value problem) numerically, with r = system in the form cAt 2(A)2 =2, i.e. write the Au = f 2. Use Thomas algorithm to solve the above linear system. Use a mesh of 100 points. You need to code this up in C, you may use triper.c from Homework 9. 3. Plot the numerical solution u(x, t) for t = 0.5, 1, 1.5, 2.5. You can use MATLAB or any other plotting software of choice. Compare the numerical solution with the exact solution at t=2.5. [Exact solution: 4 sin (x)ect 2 sin (3x)e-9ct + sin (10x)e-100ct] 4. Perform a Von-Neumann stability analysis for the scheme and comment on stability properties. 5. State the Lax-equivalence theorem. Is this scheme convergent?