Question: Consider the plane-wall problem given by at where t is temperature, 0 is time. Boundary conditions are Initial condition is : k 2t x

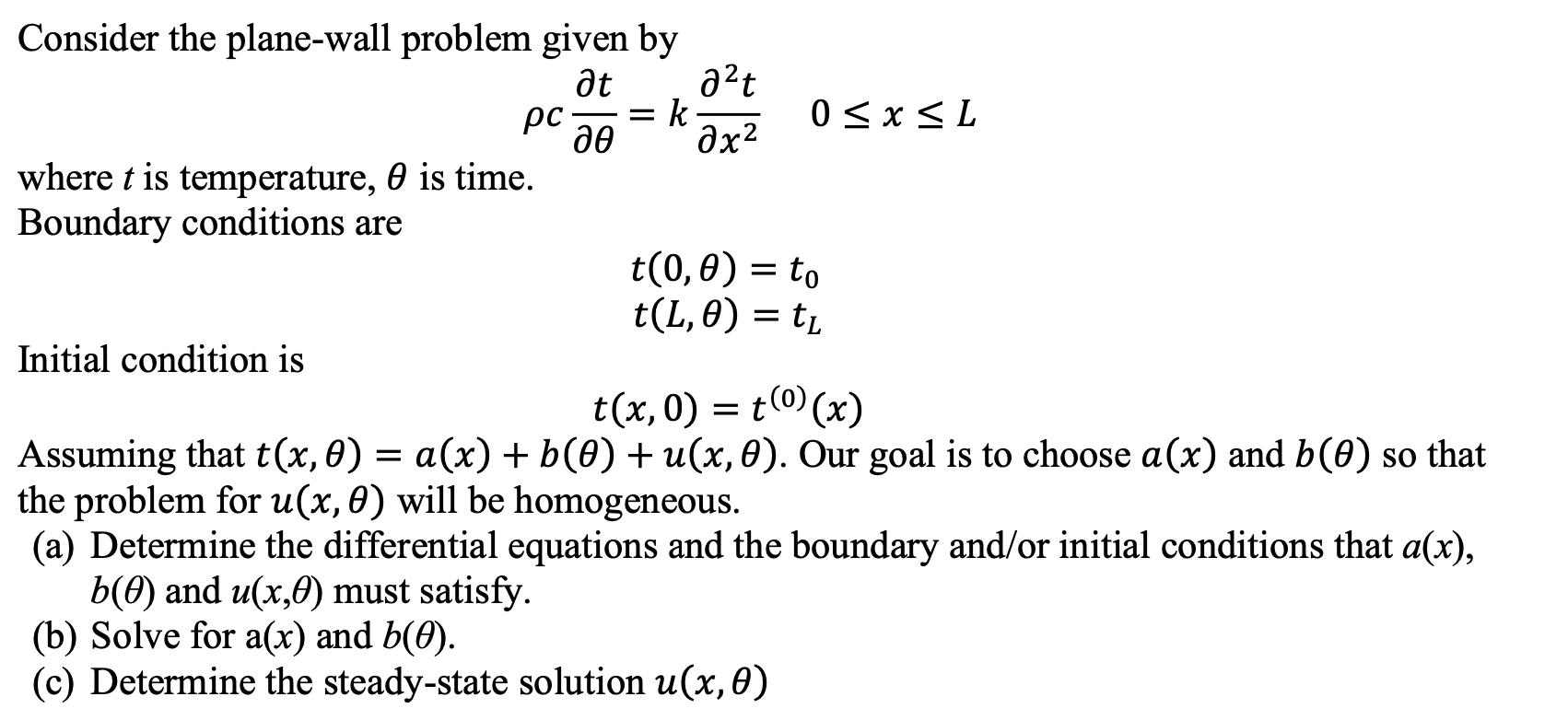
Consider the plane-wall problem given by at where t is temperature, 0 is time. Boundary conditions are Initial condition is : k 2t x 0xL t(0,0) = to t(L, 0) = t t(x,0) = t(0)(x) Assuming that t(x, 0) = a(x) + b(0) + u(x, 0). Our goal is to choose a (x) and b(0) so that the problem for u(x, 0) will be homogeneous. (a) Determine the differential equations and the boundary and/or initial conditions that a(x), b(0) and u(x,0) must satisfy. (b) Solve for a(x) and b(0). (c) Determine the steady-state solution u(x, 0)

Step by Step Solution

★★★★★

3.47 Rating (157 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

SOLUTION a To make the problem for ux0 homogeneous we need to eliminate the terms involving ax and b... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related General Management Questions!

When the bargaining process breaks down between union representatives and company management, union members occasionally will vote to stop working until demands are met; if the majority wins,...

Managing Scope Changes Case Study Scope changes on a project can occur regardless of how well the project is planned or executed. Scope changes can be the result of something that was omitted during...

The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...

Consider the plane wall, long cylinder, and sphere shown schematically, each with the same characteristic length a. thermal conductivity k, and uniform volumetric energy generation rate q. (a) On the...

Consider the situation in Problem 58 with a ladder of length L and mass M. Find the ratio of your speed as you hit the ground if you hang on to the ladder to your speed if you immediately step off as...

Suppose that log(y0 follows a linear model with a linear form of heteroskedasticity. We write this as so that, conditional on x, u has a normal distribution with mean (and median) zero but with...

Consider the series solution, Equation 5.39, for the plane wall with convection. Calculate mid plane (x* = 0) and surface (x* = 1) temperatures * for Fo = 0.1 and I, using Bi = 0.1, 1, and 10....

Consider the one-dimensional wall shown in the sketch, which is initially at a uniform temperature T j and is suddenly subjected to the convection boundary condition with a fluid at T . For a...

Consider the composite wall of Problem 2.53. In part (d), you are asked to sketch the temperature histories at x = 0, L during the transient period between cases 2 and 3. Calculate and plot these...

Consider the cantilever beam problem shown previously in Exercise 8.2 with no axial force (N =0). Assume a plane stress anisotropic model given by Hookes law (11.5.1) and governed by the Airy stress...

Which of the following commands shows the current default route without performing DNS lookups on the IP address ( es ) involved? Question 4 options: netstat - rn netstat - n netstat - r netstat f

Flip a coin 100 times and set X = # of heads. (a) How many sequences of 100 coin flips include exactly 50 heads? (b) What is the probability of a single sequence of 100 flips with exactly 50 heads?...

Under ERISA, which is the federal law that governs private pension plans, employees typcially must become \"vested\' in their benefits within how many years?

You've been hired as an organizational behavior consultant. An organization is grappling with an environment that has n ineffective culture. Growth is stagnant or declining. People are leaving their...

a. Government declares a compulsory festival bonus of 10000/- to all workers working in the country. Explain the likely impact on the demand for refrigerators in the country?

Imagining that you are a human resources manager, write a email to all those who report to you informing them that late arrivals, long lunches, and overly casual workplace clothes and behavior will...

On July 10, 2020, Pearl Ltd. sold GPS systems to retailers on account for a selling price of $840,000 (cost $672,000). Pearl grants the right to return systems that do not sell in three months...

Write a paper detailing a geographic information system (GIS) of your own design that would utilize data in an original manner.

UseWielandt deflation and the results of Exercise 7 to approximate the second most dominant eigenvalue of the matrices in Exercise 1. Iterate until a tolerance of 104 is achieved or until the number...

Use the Inverse Power method to determine, to within 105, the eigenvectors of the matrices in Exercise 2. In exercise a. b. c. d. 2 0 1 -12 0 -2 3 023 142 00024 00242 02420 24200 42000 00013 00031...

Gauss-Jordan Method: This method is described as follows. Use the ith equation to eliminate not only xi from the equations Ei+1, Ei+2, . . . , En, as was done in the Gaussian elimination method, but...

Suppose that a sample of size 8 is chosen randomly from a normally distrib uted population with mean 24 and variance 9. The standardized value cor responding to each observed value is computed by...

Show that the fact that [N/ (N l)]s2 is an unbiased estimator of a2 and that iVs2/a2 is a chi-square variable implies that the expectation of a chi-square random variable with IV 1 degrees of...

Describe how an understanding of social psychology can help people improve their lives.