Question: Consider the space V = R equipped with the Euclidean norm || ||2 and the space W = R equipped with the norm |||||3

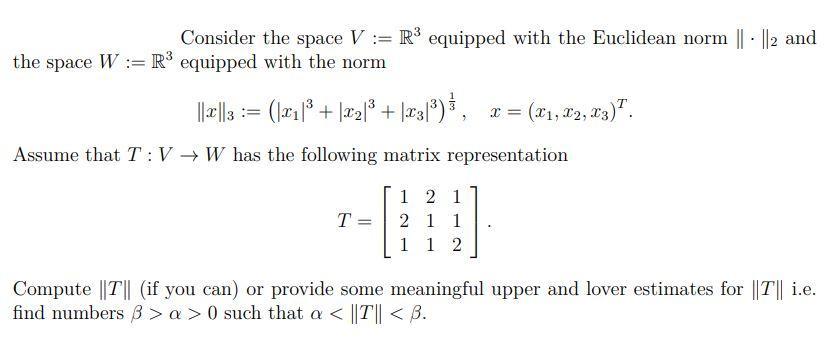
Consider the space V = R equipped with the Euclidean norm ||

Consider the space V = R equipped with the Euclidean norm || ||2 and the space W = R equipped with the norm |||||3 = (|x| + |x| + |23|) , x c = (x, x2, X3). Assume that T: VW has the following matrix representation T = 1 2 1 2 11 112 Compute ||T|| (if you can) or provide some meaningful upper and lover estimates for ||T|| i.e. find numbers > a>0 such that a < ||T||

Step by Step Solution

★★★★★

3.51 Rating (154 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The operator norm of a linear transformation TV W between two normed spaces V and W is defined as T ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

The comparative financial statements of Stargel Inc. are as follows. The market price of Stargel Inc. common stock was $119.70 on December 31, 2014. Liabilities Instructions Determine the following...

The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...

The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-6. On December 12, Irene purchased the building where her store is located. She paid...

KYC's stock price can go up by 15 percent every year, or down by 10 percent. Both outcomes are equally likely. The risk free rate is 5 percent, and the current stock price of KYC is 100. (a) Price a...

20 If log, 3 = 1.10 and log, 6 = 1.79, then log, 18 =

MATH 4140/7140 Homework 5 Due 7/21/2016 Do all problems on different paper. I will not grade anything written on this page. Show your work. Answers without work will be given little or no credit. 1....

10. . Consider a vector v R. Describe geometrically the effect of multiplying v by a scalar. Include in your answer the special cases where the scalar is 1, 0, and a negative number. Given the system...

Please solve all parts as best as you can. For A), I typed this out which might make more sense (inform me if it does not): For the "W" mentioned above, that is: ***Note: This "W" is a vector space...

Please Help Test the set S= { w, x, y, z} of the following 4 vectors to identify a subset U of 3 of them that will be sufficient to span the Euclidean space R'. To prove that your selection is...

1. Show that the function from G to G given by a > a 1 is an isomorphism iff G is abelian. 2. Determine if the following statements are TRUE or FALSE. Give reasons for your answers in each case. a)...

CNY Furniture manufactures two kinds of coffee tables. The first one is a larger table, called Adirondack. Each Adirondack has six table legs and a tabletop of 18 square feet. The second one is a...

Nick Jones, a 30-year-old single taxpayer, is involved in three partnerships; one as a general partner and two as a limited partner (Schedule K-1s provided in Canvas Files). Nick has no other source...

Name the followingcompounds: NH2 (b) (a) (c) -NHCH2CH3 -CH2CH2NH2 Br Br (e) (f) H2NCH2CH2CH2CN (d) C N-CH2CH2CH3 CH

An owner of a nursing facility opened the facility ten years ago and immediately incorporated it as a separate legal entity. The owner has always been careful to treat all income of the facility as...

Use your descriptions from Problem 1.2 to identify the physical state (at room temperature) of the following: (a) The air in your room: (b) Tablets in a bottle of vitamins; (c) Sugar in a packet.

How Many Virus Attacks Have Occurred This Month? Using some website resource, such as www.f-secure.com, look up recent computer virus outbreaks.

Part A Use the data matrix below to draft at least three alternate phylogenetic trees. Presence or Absence of Hox Genes* Hox1 Hox2 Hox3 Hox4 Hox12 Mouse 1 1 1 1 1 Snail 1 1 1 1 0 Flatworm 1 1 1 1 0...

What is an employee stock option plan (ESOP)? How is an ESOP used to buy out a venture?

When alkene 1 was treated with HBr, two alkyl halides (A and B) were isolated. a. With aid of a mechanism show how A and B are formed under the above reaction conditions. b. Which of two products...

Potential problem: You met with Go - Go's audit committee, which consists of the corporate controller, treasurer, financial vice president, and budget director.

The balance factor of an internal position p of a proper binary tree is the difference between the heights of the right and left subtrees of p. Show how to specialize the Euler tour traversal of...

In our AVL implementation, each node stores the height of its subtree, which is an arbitrarily large integer. The space usage for an AVL tree can be reduced by instead storing the balance factor of a...

Give an efficient algorithm for determining if a pattern P is a subsequence (not substring) of a text T. What is the running time of your algorithm?

In an article titled Childhood Pastimes Are Increasingly Moving Indoors, Dennis Cauchon asserts that there have been huge declines in spontaneous outdoor activities such as bike riding, swimming, and...

A marketing research firm is interested in determining whether there is a difference between the proportion of households in Chicago and the proportion of households in Milwaukee who purchase...

Suppose, as part of a national study of economic competitiveness, a marketing research firm randomly sampled 200 adults between the ages of 27 and 35 living in metropolitan Seattle and 180 adults...