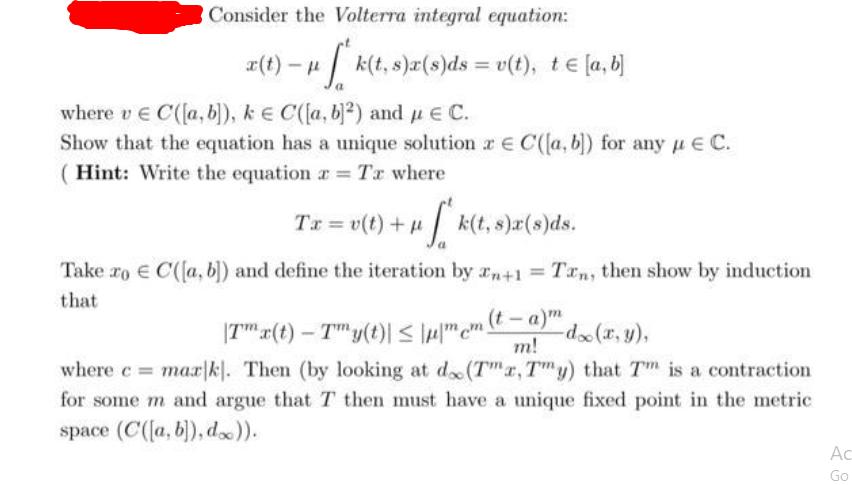
Consider the Volterra integral equation: x(t)-p pk(t,s)r(s)ds = v(t), t [a,b] a where v C([a,b]), ke C([a, b]2) and C. Show that the equation has a unique solution C([a, b]) for any C. (Hint: Write the equation = Tx where Tr = v(t) + k(t, s)x(s)ds. Take ro C([a, b]) and define the iteration by n+1 = Tan, then show by induction xn+1=1 that |T x(t) Ty(t)| |m cm (t - a)m - m! -doo (x, y), where c = max|k|. Then (by looking at do (T", Ty) that Tm is a contraction for some m and argue that T then must have a unique fixed point in the metric space (C([a, b]), d)). Ac Go