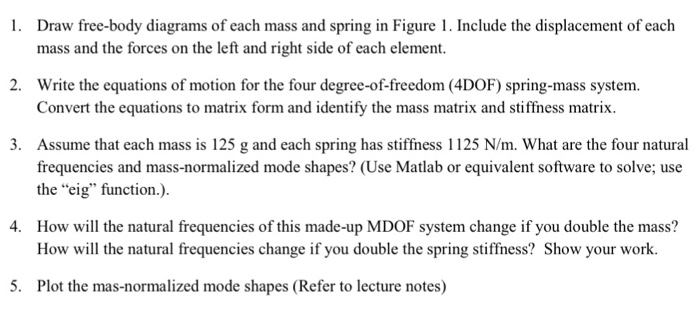
Continuous systems have an infinite number of degrees of freedom that require solution with partial differential equations. A multiple degree of freedom, or MDOF, lumped element system can approximate a continuous system and requires simpler ordinary differential equations. The equations of motion for an MDOF system are [M]+[K]=0 (1) The specific equation of motion can be derived by drawing free-body diagram for each mass and spring, and writing the Newton's law for each mass, and Hooke's law for each spring, in terms of the displacements of each mass. Natural frequencies (eigenvalues) and mode shapes (eigenvectors) of an unforced, undamped system can be determined by assuming a harmonic solution = pexp(icot), substituting into the equation of motion and solving the eigenvalue problem. Figure 1 shows an undamped four degree of freedom system fixed on both ends. Fin XI mi X2 m Figure 1. Four degree of freedom undamped system H m3 X4 m4 For the 4-DOF spring-mass system shown in Figure 1, the eigenvalue problem is ([K] - wo[M]) = 0 (2) Solving the eigenvalue problem yields the natural frequencies w, and mode shapes s (n=1,2,...4). Orthonormalization of eigenvectors with respect to the mass matrix yields mass-normalized modes: P = Pn/an where an = [M] Mass-normalized modes simplify the normal form and allow direct comparison of contributions from different modes. 1. Draw free-body diagrams of each mass and spring in Figure 1. Include the displacement of each mass and the forces on the left and right side of each element. 2. Write the equations of motion for the four degree-of-freedom (4DOF) spring-mass system. Convert the equations to matrix form and identify the mass matrix and stiffness matrix. 3. Assume that each mass is 125 g and each spring has stiffness 1125 N/m. What are the four natural frequencies and mass-normalized mode shapes? (Use Matlab or equivalent software to solve; use the "eig" function.). 4. How will the natural frequencies of this made-up MDOF system change if you double the mass? How will the natural frequencies change if you double the spring stiffness? Show your work. 5. Plot the mas-normalized mode shapes (Refer to lecture notes)