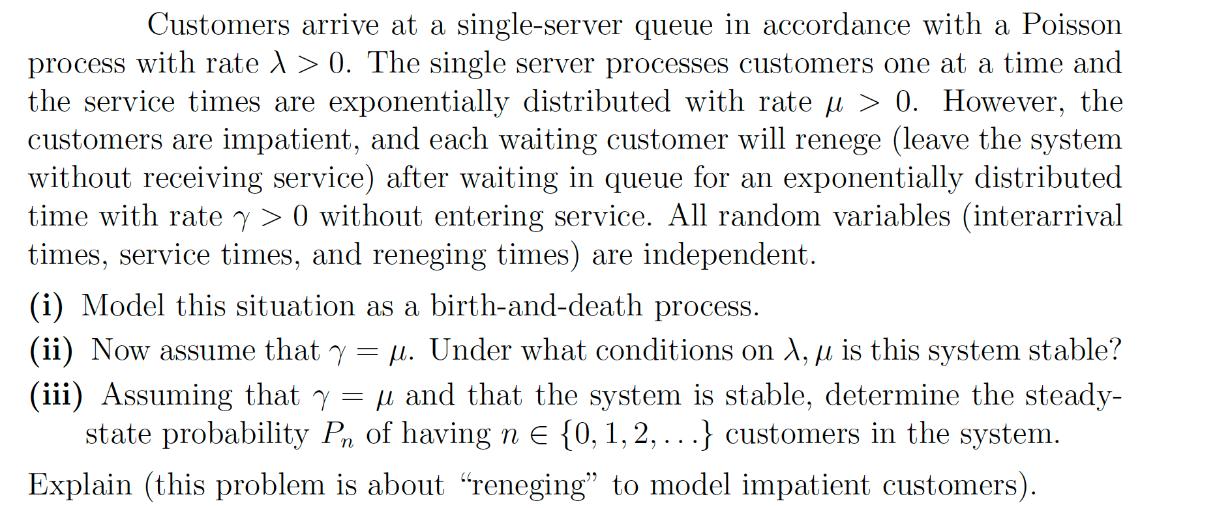
Customers arrive at a single-server queue in accordance with a Poisson process with rate > 0. The single server processes customers one at a time and the service times are exponentially distributed with rate > 0. However, the customers are impatient, and each waiting customer will renege (leave the system without receiving service) after waiting in queue for an exponentially distributed time with rate y> 0 without entering service. All random variables (interarrival times, service times, and reneging times) are independent. (i) Model this situation as a birth-and-death process. = (ii) Now assume that y . Under what conditions on A, is this system stable? (iii) Assuming that y and that the system is stable, determine the steady- state probability Pn of having n = {0, 1, 2,...} customers in the system. Explain (this problem is about "reneging" to model impatient customers).