Data was collected for 30 professional tennis players regarding their performance in Grand Slams (the four major tennis tournaments in the world). The response variableWin, expressed as a proportion ranging from 0 to 1, is believed to depend on two explanatory variables: the number of double faults and the number of aces.

The following model is estimated:

Win=?

0

+?

1

??DoubleFaults+?

2

Aces+?

Win=?0+?1???DoubleFaults?+?2Aces+?.

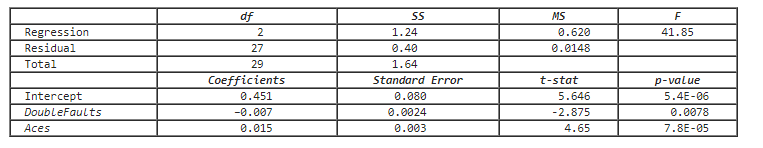
A portion of the regression results is shown in the accompanying table.

a. Predict the winning percentage for a player who had 20 double faults and five aces (enter your answer as a percentage rounded to one decimal place.)

%

b.Interpret the interceptof the estimated regression equation. Enter the coefficient as a percentage.

The predicted

(Click to select)

winning proportion

number of double faults

number of aces

is% when the number of

(Click to select)

winning proportion and number of double faults

number of double faults and aces

winning proportion and number of aces

(Click to select)

are equal to

increase by

decrease by

.

c. Interpret the slope coefficient for the variableDoubleFaults. Enter the slope coefficient as an absolute percentage value andindicatewhether it increases or decreases.

The

(Click to select)

number of double faults

winning proportion

number of aces

is expected to

(Click to select)

be equal to

decrease by

increase by

% for each additional

(Click to select)

double fault

win

ace

, while holding the

(Click to select)

number of aces

winning proportion

number of double faults

constant.

d. Calculate the standard error of the estimate(enter your answer as a percentage rounded to one decimal.)

%

e. Calculate and interpret the coefficient of determination (enter your answer as a percentage rounded to one decimal.)

% of the

(Click to select)

double faults

winning proportion

variation in winning proportion

variation in double faults

can be explained by the

(Click to select)

winning proportion and double faults

variation in winning proportion and double faults

variation in double faults and aces

number of double faults and aces

.

f. Calculate the adjustedR²(enter your answer as a percentage rounded to one decimal.)

%

df 55 MS F Regression 2 1. 24 0.620 41.85 Residual 27 0.40 0. 0148 Total 29 1.64 Coefficients Standard Error t-stat p-value Intercept 0.451 0.080 5.646 5.4E-06 DoubleFaults -0. 007 0. 0024 -2.875 3. 0078 Aces 0 . 015 0.003 4.65 7.BE-05