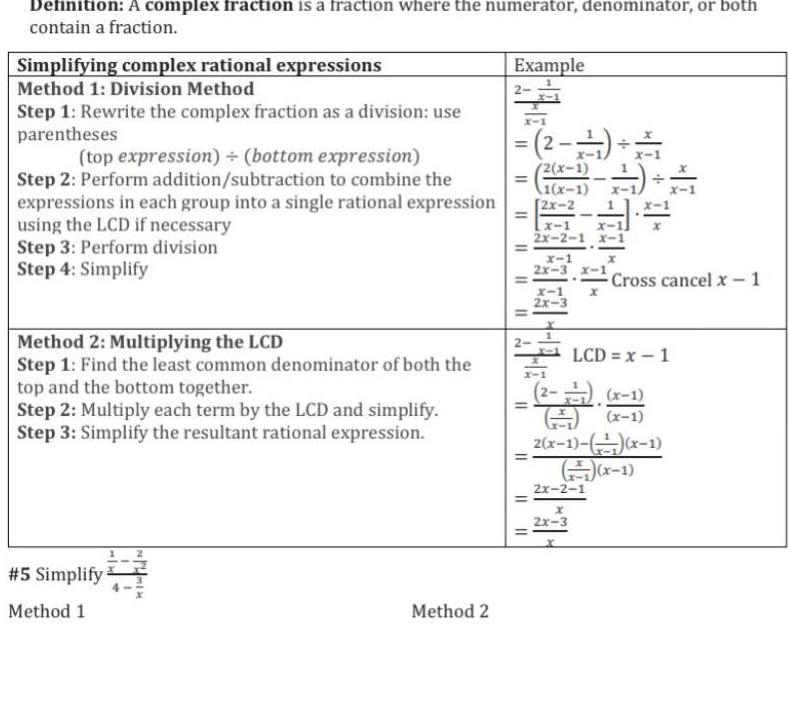
Definition: A complex fraction is a fraction where the numerator, denominator, or both contain a fraction. Simplifying complex rational expressions Method 1: Division Method Step 1: Rewrite the complex fraction as a division: use parentheses (top expression) + (bottom expression) Step 2: Perform addition/subtraction to combine the expressions in each group into a single rational expression using the LCD if necessary Step 3: Perform division Step 4: Simplify Example 2- = (2-1) = (2(x-1) - 1(x-1) x-1 x-1 2x-21.x-1 x-1 x-1 2x-2-1 x- x-1 2x-3x-1 x-1 2x-3 x Cross cancel x-1 Method 2: Multiplying the LCD Step 1: Find the least common denominator of both the top and the bottom together. Step 2: Multiply each term by the LCD and simplify. Step 3: Simplify the resultant rational expression. #5 Simplify Method 1 Method 2 == x-1 LCD =x-1 (2-)(x-1) (x-1) 2(x-1)-((x-1) (x)(x-1) 2x-2-1 === x 2x-3 =