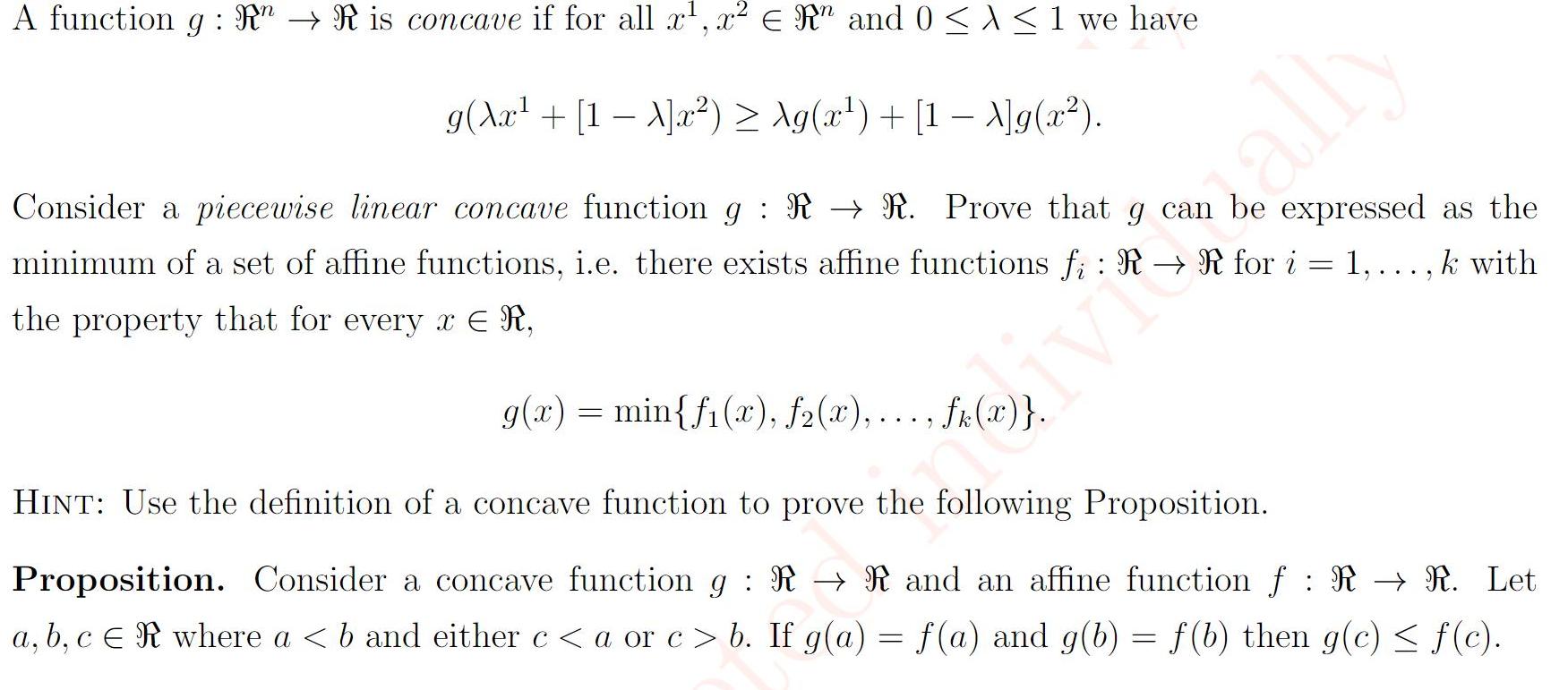
A function g : R" R is concave if for all x, x? E R" and 0 \g(x') + [1 A]g(x?). - Consider a piecewise linear concave function g : R R. Prove that g can be expressed as the ally minimum of a set of affine functions, i.e. there exists affine functions f; : R R for i the property that for every x E R, 1,..., k with g() = min{fi(x), f2(x), ... , fr(x)}. HINT: Use the definition of a concave function to prove the following Proposition. Proposition. Consider a concave function g: R and an affine function f: R R. Let a, b, c E R where a b. If g(a) = f(a) and g(b) = f(b) then g(c) < f(c).