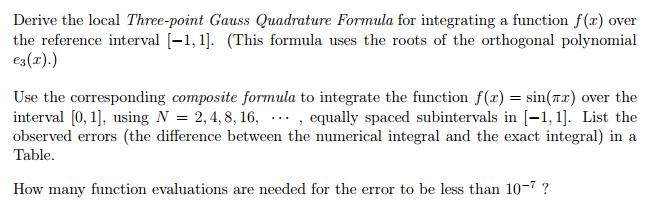
Derive the local Three-point Gauss Quadrature Formula for integrating a function f(x) over the reference interval [-1, 1]. (This formula uses the roots of the orthogonal polynomial 3(x).) Use the corresponding composite formula to integrate the function f(x) = sin(x) over the interval [0, 1], using N = 2,4,8, 16, ..., equally spaced subintervals in [-1,1]. List the observed errors (the difference between the numerical integral and the exact integral) in a Table. How many function evaluations are needed for the error to be less than 10-7 ?