Derive the order of the error with respect to the sin and cosine approximations. sin(x)=x-x/3!+x/5!-x'/7!+... cos(x)...

   

Transcribed Image Text:

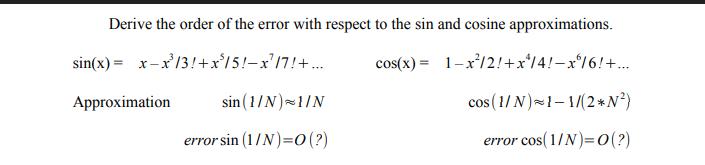
Derive the order of the error with respect to the sin and cosine approximations. sin(x)=x-x/3!+x/5!-x'/7!+... cos(x) = 1-x/2!+x/4!-x16!+.... Approximation sin (1/N)=1/N error sin (1/N)=0 (?) cos (1/N) 1-1/(2*N2) error cos(1/N)=0(?) Derive the order of the error with respect to the sin and cosine approximations. sin(x)=x-x/3!+x/5!-x'/7!+... cos(x) = 1-x/2!+x/4!-x16!+.... Approximation sin (1/N)=1/N error sin (1/N)=0 (?) cos (1/N) 1-1/(2*N2) error cos(1/N)=0(?)

Answer rating: 100% (QA)

Lets start by analyzing the sin approximation The Taylor series expansion for sinx is given by sinx ... View the full answer