Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Design a feedback control law to linearize the system. B ) linearize the given system using backstepping integrator. x 1 = x 2 x 2
Design a feedback control law to linearize the system. Blinearize the given system using backstepping integrator.
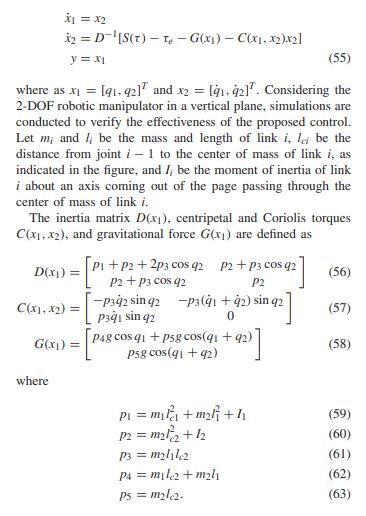
where as and Considering the
DOF robotic manipulator in a vertical plane, simulations are
conducted to verify the effectiveness of the proposed control.
Let and be the mass and length of link be the
distance from joint to the center of mass of link as
indicated in the figure, and be the moment of inertia of link
i about an axis coming out of the page passing through the
center of mass of link
The inertia matrix centripetal and Coriolis torques
and gravitational force are defined as
gcos
where
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


