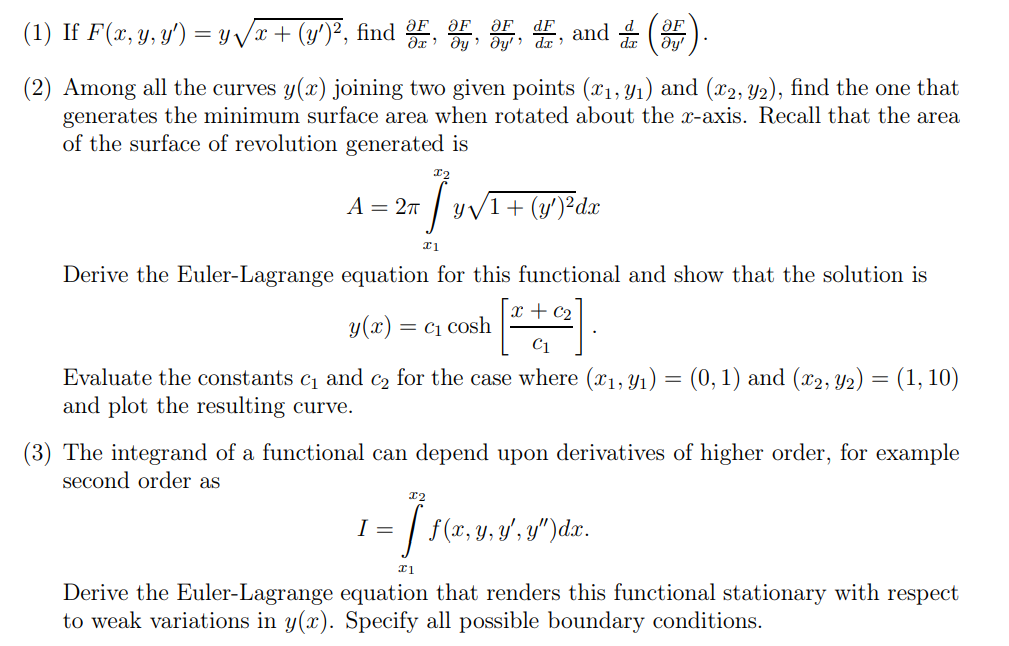
dF (1) If F(x, y, y') = yx + (y'), find F, F, F, F, and 1 (F). (2) Among all the curves y(x) joining two given points (x1, y) and (x2, y2), find the one that generates the minimum surface area when rotated about the x-axis. Recall that the area of the surface of revolution generated is A = 2T x2 y1+ (y')dx x1 Derive the Euler-Lagrange equation for this functional and show that the solution is y(x) = C cosh x + C2 C1 Evaluate the constants c and 2 for the case where (x1, 1) = (0, 1) and (x2, y2) = (1, 10) and plot the resulting curve. (3) The integrand of a functional can depend upon derivatives of higher order, for example second order as x2 I = = [[(x, y, ' y)dx. x1 Derive the Euler-Lagrange equation that renders this functional stationary with respect to weak variations in y(x). Specify all possible boundary conditions.