Question: Do c and d Do b, d, e Do b Apply De Morgan's law to each expression to obtain an equivalent expression in which each

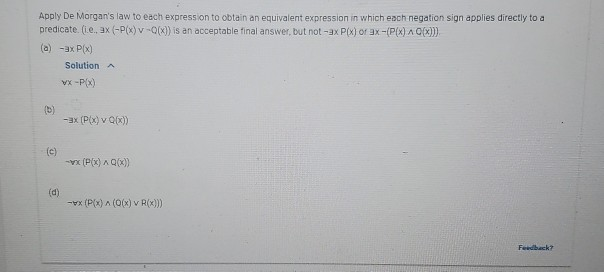
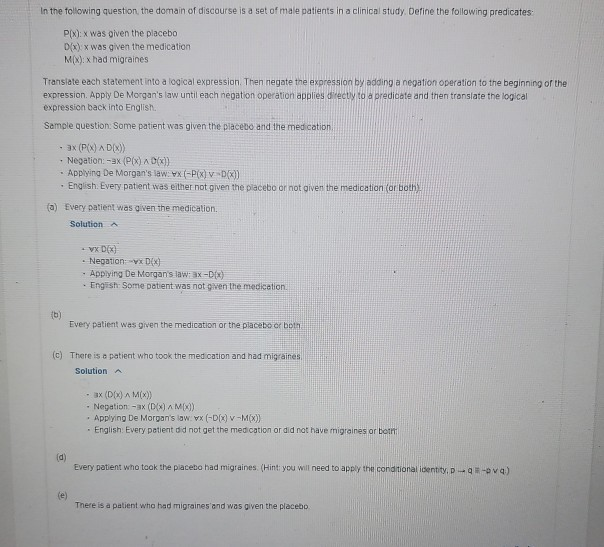
Do c and d Do b, d, e Do b Apply

Do c and d

De Morgan's law to each expression to obtain an equivalent expression in

Do b, d, e

which each negation sign applies directly to a predicate. (i.e., 3x (-P(x)

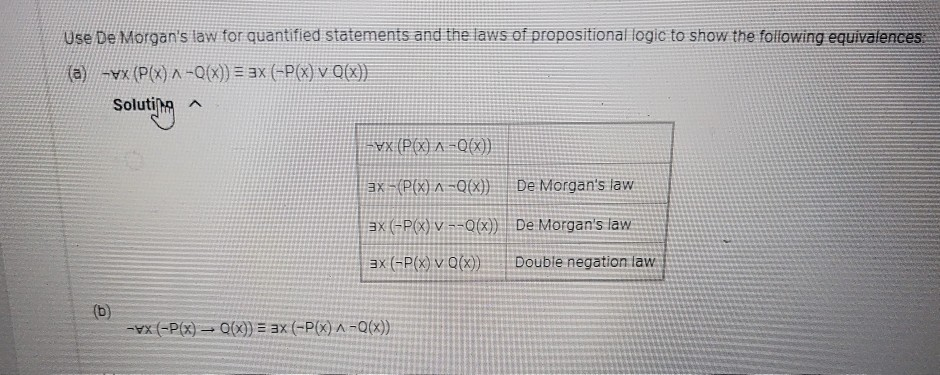
Do b

Apply De Morgan's law to each expression to obtain an equivalent expression in which each negation sign applies directly to a predicate. (i.e., 3x (-P(x) v -Q(x)) is an acceptable final answer, but not -ax P(x) or 3x-(P(x) A Q(x))). (a) -ax P(x) SolutionA Vx -P(x) (b) -3x (P(x) v Q(x) (e) -x (P(x) A Q(x) (d) -vx (P(x) A (O(x) v R(x)) Feedback? In the following question the domain of discourse is a set of male patients in a clinical study. Define the following predicates: P(x): X was given the placebo D(x): x was given the medication M(X): x had migraines Transiate each statement into a logical expression. Then negate the expression by adding a negation operation to the beginning of the expression. Apply De Morgan's law until each negation operation applies directly to a predicate and then translate the logical expression back into English. Sample question: Some patient was given the placebo and the medication. - ax (P(X) A D(X) Negation: -ax (P(x) A D(x)) - Applying De Morgan's law: VX (-P(x) v D(x)) English. Every patient was either not given the placebo or not given the medication (or both) a) Every patient was given the medication. Solution A vx D(x) Negation: vx D(X) Applying De Morgans law: ax -Dx) Engish: Some patient was not given the medication. (b) Every patient wes given the medication or the placebo or botn (c) There is a patient who took the medication and had migraines Solution ax (D(x) A M(x) - Negation -ax (D(x) A M(X) Applying De Morgan's law: vx (-D(x) v -M(X) English: Every petient did not get the medication or did not have migraines or botn (d) Every patient who took the piacebo had migraines. (Hint: you will need to apply the conditional identity, p-aE-va) (e) There is a patient who had migraines and was given the placebo Use De Morgan's law for quantified statements and the laws of propositional logic to show the following equivalences (a) -vx (P(x) ^ -Q(x)) = 3x (-P(x) v Q(x)) solutg Vx (P(x) a ~Q(x)} ax -(P(x) ^ ~Q(x)) De Morgan's law ax (-P(x) v --Q(x)) De Morgan's law ax (P(x) v Q(x)) Double negation law (b) Vx (-P(x) Q(x)) = 3x (-P(x) ^ Q(x))

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Databases Questions!

Apply De Morgan's law o each expression to obtain an equivalent expression in which each negation sign applies directly to a predicate (i.e., thereexists x(P(x) v Q(x)) is an acceptable final answer,...

Apply De Morgan's law to each expression to obtain an equivalent expression in which each negation sign applies directly to a predicate. For example, is an acceptable final answer, but not or ( a ) (...

Apply De Morgan's law to each expression to obtain an equivalent expression in which each negation sign applies directly to a predicate. For example, Ex ( P ( x ) V Q ( x ) ) is an acceptable final...

Apply De Morgan's law to the expression to obtain an equivalent expression in which the negation sign applies directly to a predicate. For example, x ( P ( x ) Q ( x ) ) is an acceptable final...

Here is a copy of Schedule D and Form 8949 for completing the required assignment,Instructions for all IRS forms are a great resource and can be found at www . irs .gov. Here are links to...

True and False-l point 1. Much of marrial accounting is directed at gathering useful information about costs for planning and control decisions 2. Control is the process of setting goals and...

a) If you have the flu, you miss the final examination. b) You pass the course if and only if you do not miss the final examination. c) If you miss the final examination, then you will not pass the...

Show work/explanation where indicated. Answers without any work may earn little, if any, credit. You may type or write your work in your copy of the exam, or if you prefer, create a document...

The current stock price is $80. The stock does not pay dividends. All contracts mature in one year. The annual effective rate of interest is 3.5%, F(0,7) (toward price) -82.8. Tony short-sells two...

The return on assets for Tresh Corporation is 7.6%. During the same year Treshs return on common stockholders equity is 12.8%.What is the explanation for the difference in the two rates?

Which one of the following is an example of a financial asset? a . Inventory b . Loans receivable c . Property, Plant and Equipment d . Trade payable

5 a. Assume the returns of a stock for the previous five years are as follows: 7%, 6%, 5%, - 4% and -3%? What is the historical standard deviation of the returns of this stock? If the returns are...

1. Describe the two main philosophical approaches underlying management employee relations and comment on how these approaches affect employee involvement initiatives.

3. Describe the principal employee involvement categories and practices.

describe European Union initiatives relating to employee rights to information and consultation