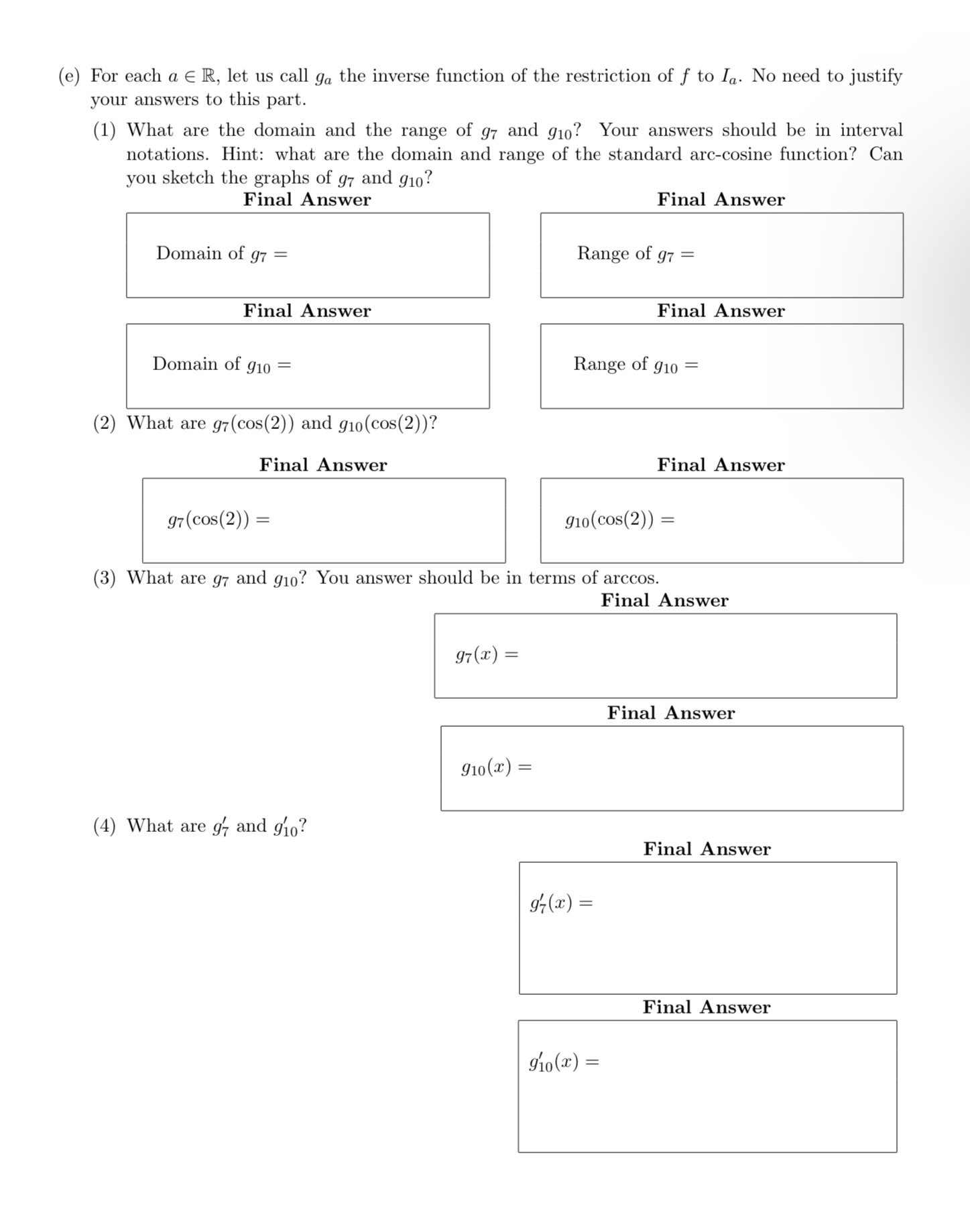
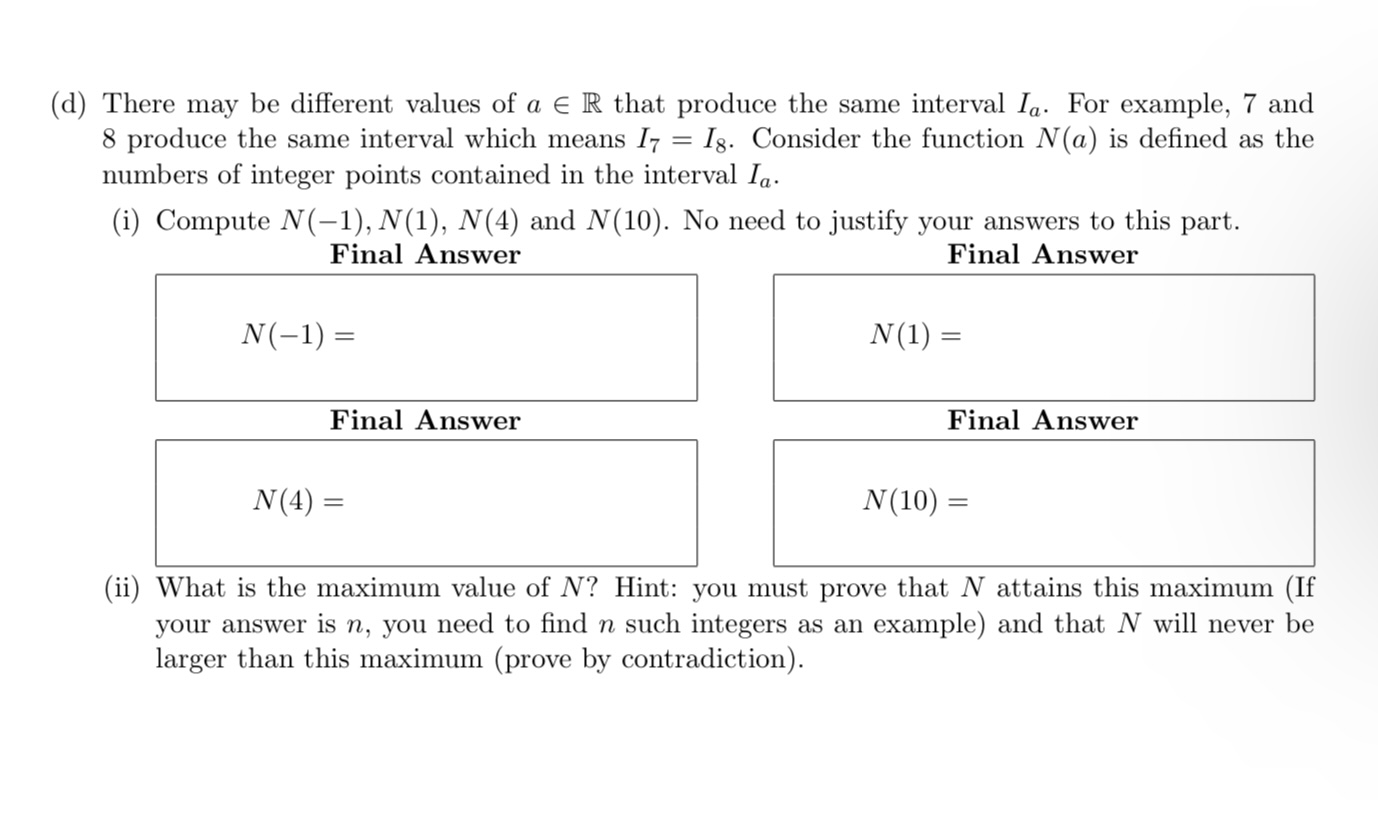
(e) For each a R, let us call ga the inverse function of the restriction of f to Ia. No need to justify your answers to this part. (1) What are the domain and the range of 97 and 910? Your answers should be in interval notations. Hint: what are the domain and range of the standard arc-cosine function? Can you sketch the graphs of 97 and 910? Final Answer Domain of 97 = Final Answer Domain of 910 = (2) What are g7 (cos(2)) and g10 (cos(2))? Final Answer 97(cos (2)) = (4) What are g7 and 910? 97(x) = 910 (x) = Range of 97 = Final Answer (3) What are g7 and 910? You answer should be in terms of arccos. Range of 910 = 910 (cos(2)) = 97(x) = 910 (x) = nal Answer Final Answer Final Answer Final Answer Final Answer Final Answer (h) For each a A, compute ga (f(2022)) and f(ga (2022)). Or if they are not defined, explain why. (d) There may be different values of a R that produce the same interval Ia. For example, 7 and I8. Consider the function N(a) is defined as the 8 produce the same interval which means 17 numbers of integer points contained in the interval la. (i) Compute N(1), N(1), N(4) and N(10). No need to justify your answers to this part. Final Answer Final Answer N(-1) = Final Answer = N(4) = N(1) = Final Answer N(10) = (ii) What is the maximum value of N? Hint: you must prove that N attains this maximum (If your answer is n, you need to find n such integers as an example) and that N will never be larger than this maximum (prove by contradiction). = (f) Construct a set ACR such that any two different elements of A produce different intervals. Moreover, all possible interval Ia are produced by some element in A: Vb R for which the interval It is well defined, there exists exactly one a A such that la Ib. Hint: you need to prove your answer is true with three steps: 1) for any a A, the interval la is well-defined; 2) two different elements of A produce different intervals; 3) any interval Ia is produced by an element of A. (g) Now let a A. What are the domain and the range of g? Justify your answers. Sketch its graph (labelling the axes properly). 3. (Note: Before you attempt this problem, solve Problem 10 of Practice problems for Unit 4 on Quercus. Otherwise, you may find this question difficult.) Let us consider the function f : R R defined by f(x) = cos(x). It is not one-to-one. Hint: the graph of f(x) may help you solve this question. (iii) What is the minimum value of N? Hint: you must prove that N attains this minimum and that N will never be less than this minimum.