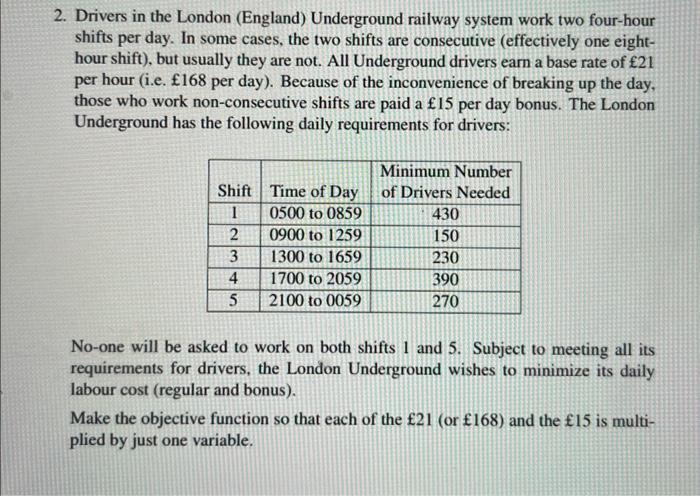
Each question in Assignment 2 is worth 20 marks. For each question, do the following: (a) Formulate a model for the problem either in LINGO syntax or an Excel file. 1 For each model: (i) Write your name and student number. (ii) Write the definitions of all the variables. (This is the same as what was given for Q1; make your own variable definitions for the other questions.) (iii) Write the objective function. (iv) Write the constraints with comments to explain their purpose. 2 (v) The non-negativity restrictions are to be omitted. 3 (vi) LINGO only: Every variable which must be integer must be so declared using an @GIN command. This is followed by an END command. Copy-and-paste the LINGO file into the Word or pdf submission document. (vii) Excel only: The Solver Parameters box must be filled in, including an int declaration for each integer variable. Copy-and-paste the Excel file into the Word or pdf submission document, and include a screenshot of the Solver Parameters box into this document. (b) Solve the model, and copy-and-paste either the LINGO solution file or the Excel Solver Answer Report into the Word or pdf submission document. (c) State the solution in words in the Word or pdf submission document. When you attempt to solve these models, you might receive a message about the solution being infeasible. While this is a theoretical possibility, it won't happen for any of hese questions. It would mean that a mistake has been made in formulating the model. 2. Drivers in the London (England) Underground railway system work two four-hour shifts per day. In some cases, the two shifts are consecutive (effectively one eighthour shift), but usually they are not. All Underground drivers earn a base rate of 21 per hour (i.e. 168 per day). Because of the inconvenience of breaking up the day, those who work non-consecutive shifts are paid a 15 per day bonus. The London Underground has the following daily requirements for drivers: No-one will be asked to work on both shifts 1 and 5 . Subject to meeting all its requirements for drivers, the London Underground wishes to minimize its daily labour cost (regular and bonus). Make the objective function so that each of the 21 (or 168 ) and the 15 is multiplied by just one variable