Equations of motion for MDOF systems hold key dynamic information that we are interested in: modal properties, i.e. natural frequencies and mode shapes. Solution of these equations completely describes the motion of the system (given initial conditions)

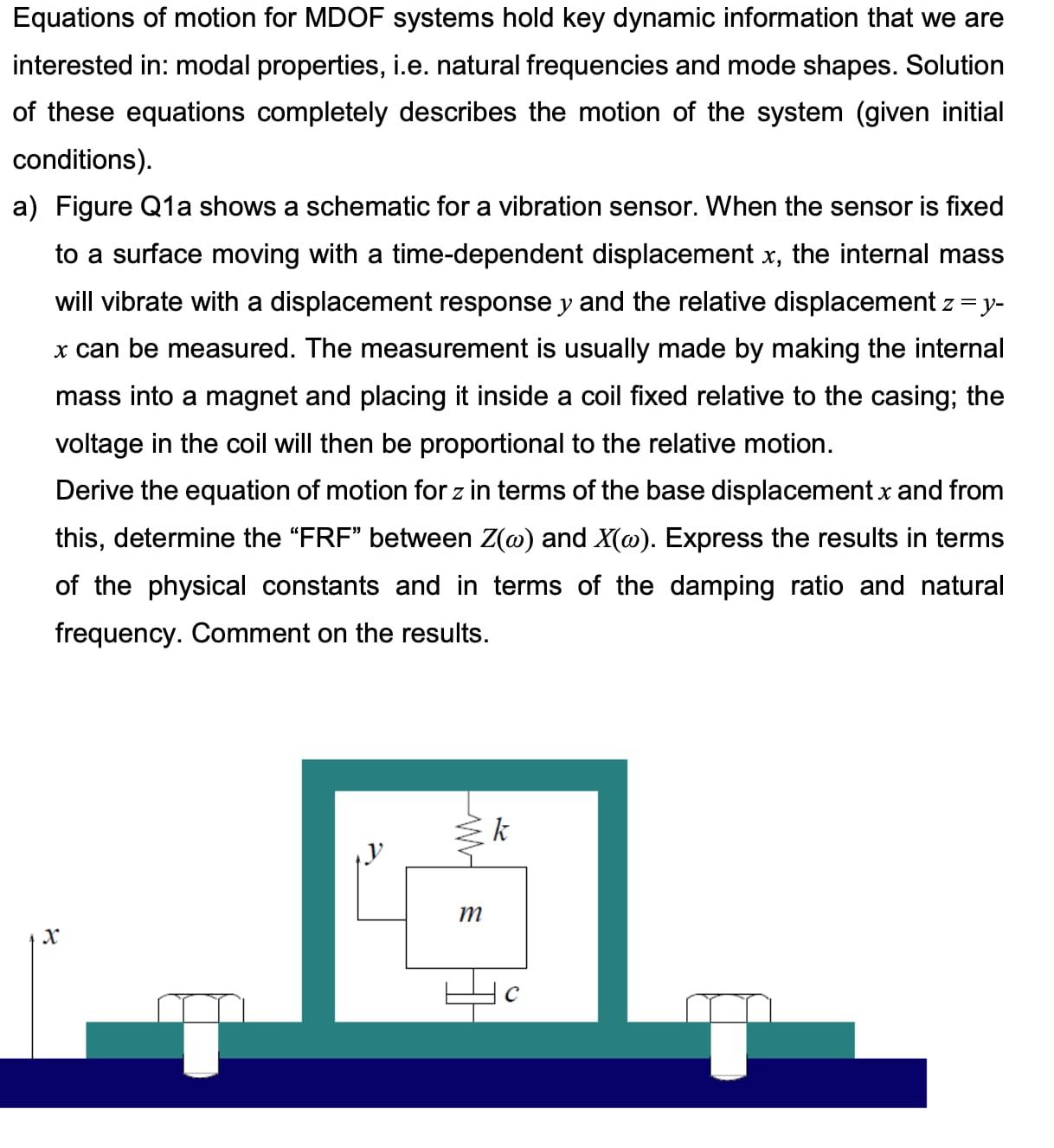
a) Figure Q1a shows a schematic for a vibration sensor. When the sensor is fixed to a surface moving with a time-dependent displacement x, the internal mass will vibrate with a displacement response y and the relative displacement z=y x can be measured. The measurement is usually made by making the internal mass into a magnet and placing it inside a coil fixed relative to the casing; the voltage in the coil will then be proportional to the relative motion. Derive the equation of motion for z in terms of the base displacement x and from

this, determine the "FRF" between Z(@) and X(w). Express the results in terms

of the physical constants and in terms of the damping ratio and natural

frequency. Comment on the results.

Equations of motion for MDOF systems hold key dynamic information that we are interested in: modal properties, i.e. natural frequencies and mode shapes. Solution of these equations completely describes the motion of the system (given initial conditions). a) Figure Q1a shows a schematic for a vibration sensor. When the sensor is fixed to a surface moving with a time-dependent displacement x, the internal mass will vibrate with a displacement response y and the relative displacement z = y- x can be measured. The measurement is usually made by making the internal mass into a magnet and placing it inside a coil fixed relative to the casing; the voltage in the coil will then be proportional to the relative motion. Derive the equation of motion for z in terms of the base displacement x and from this, determine the "FRF" between Z(w) and X(w). Express the results in terms of the physical constants and in terms of the damping ratio and natural frequency. Comment on the results. m k