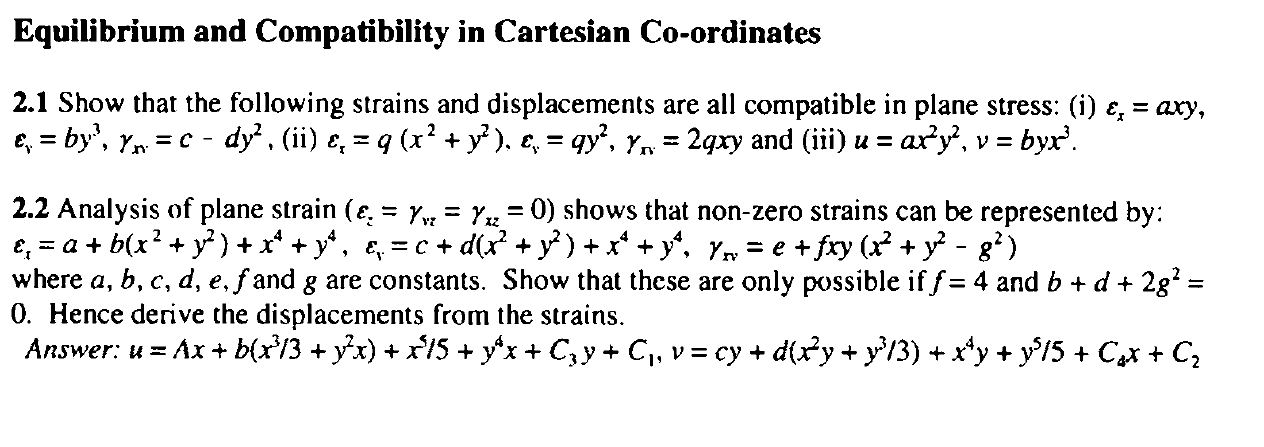
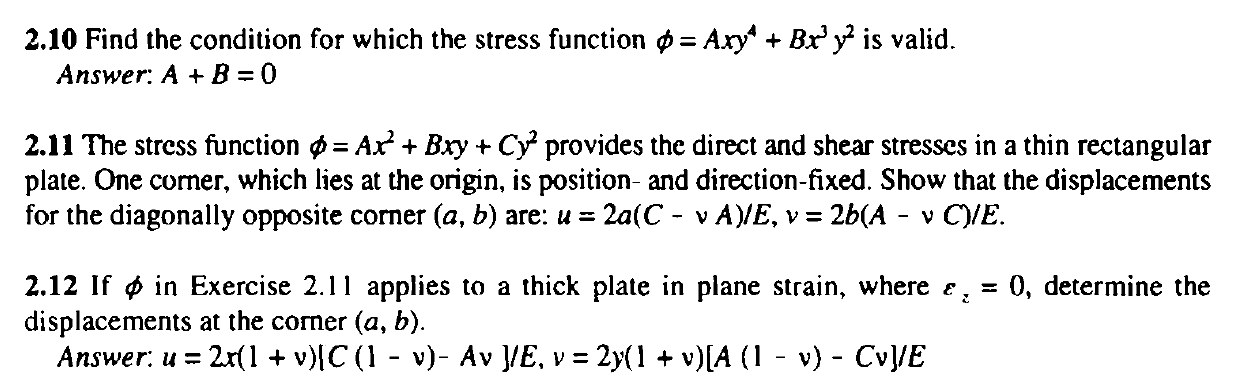
Equilibrium and Compatibility in Cartesian Co-ordinates 2.1 Show that the following strains and displacements are all compatible in plane stress: (i) , = axy, = by, Yn = c - dy, (ii) , = q (x + y ), = qy, y = 2qxy and (iii) u = : axy, v = byx. 2.2 Analysis of plane strain ( = Y = Yxz = 0) shows that non-zero strains can be represented by: = a + b (x + y ) + x + y, & = c + d(x + y ) + x + y, Y = e + fxy (x + y - g) where a, b, c, d, e, and g are constants. Show that these are only possible if f = 4 and b + d + 2g : 0. Hence derive the displacements from the strains. = Answer: u = Ax + b(x/3 + yx) + x15 + y x + Czy + C, v = cy + d(xy + y/3) + xy + y/5 + Cx + C 2.5 The stress system in a plate consists of direct tensile stresses = cyx, o = cy/3 together with a shear stress In Determine Answer: T = - cxy2 2.7 A square plate of side length a and with sides along the co-ordinate axes is fixed in position at the origin and with the side along the y-axis fixed in direction ( ulay = 0). If the stresses in the plate are = Ayla, = A with a consistent value of shear stress, find expressions for this shear stress and the general displacements. What are the displacements existing at the corner (a,a)? (IC) Answer: = K, u = Axy/(aE) - v Ax/E, v =, = Ay/E v Ay/(2aE) + 2Kx(1 + v)/E - Ax/(2aE) and at (a, a), u = Aa(1 v )/E, v = Aa(1 v )/(2E) + 2Ka(1 + v )/E 2.5 The stress system in a plate consists of direct tensile stresses = cyx, o = cy/3 together with a shear stress In Answer: T = Determine cry In 2.10 Find the condition for which the stress function = Axy + Bx y is valid. Answer: A + B = 0 2.11 The stress function = Ax + Bxy + Cy provides the direct and shear stresses in a thin rectangular plate. One corner, which lies at the origin, is position- and direction-fixed. Show that the displacements for the diagonally opposite corner (a, b) are: u = 2a(C - v A)/E, v = = 2b(A v C)/E. 2.12 If in Exercise 2.11 applies to a thick plate in plane strain, where & = 0, determine the displacements at the corner (a, b). - Answer: u = 2x(1 + v)[C (1 v)- Av ]/E, v = 2y(1 + v)[A (1 - v) - Cv]/E 2.31 For a particular plane strain problem the strain-displacement equations in cylindrical co-ordinates (r,8, 2) are , = du/dr, &,= ulr, ,= Y,, = Y = = 0. Show that the appropriate compatibility equation in terms of stress is rv do,/dr-r(1 - v )do/dr + , 0,= 0. State the nature of a problem represented by the above equations. (CEI) 2.32 A thick-walled cylinder with inner and outer radii r, and r, respectively is pressurised both internally (p.) and externally (p.). Find the expressions for the constants A and C in the corresponding stress function = A ln r + CP. Answer: A = (p, - p,) r,/[(r,/r;) - 1], 2C = [p; - p(r,/ r;) ]/[(r,/ r;) - 1]