Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Euler's Totient function (or Euler's Phi function) is a function that accepts a positive integer n and returns how many positive integers less than n

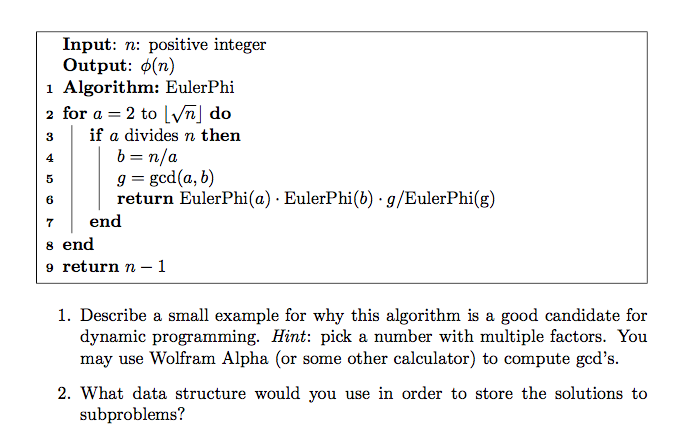
Euler's Totient function (or Euler's Phi function) is a function that accepts a positive integer n and returns how many positive integers less than n are relatively prime to n. at is, they have greatest common divisor of 1 with n As an example, consider computing p(12). We can see from the following table that only 1, 5, 7, and 11 are relatively prime to 12, so b(12) 4. gcd(r, 12) 10 11 The naive EulerPhi algorithm below computes Euler's Phi recursively based on the following recurrence if n is prime or 1 (b) gcd (a,b where a is a factor of n and b n/a 52, $(gcd(a,b)
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


