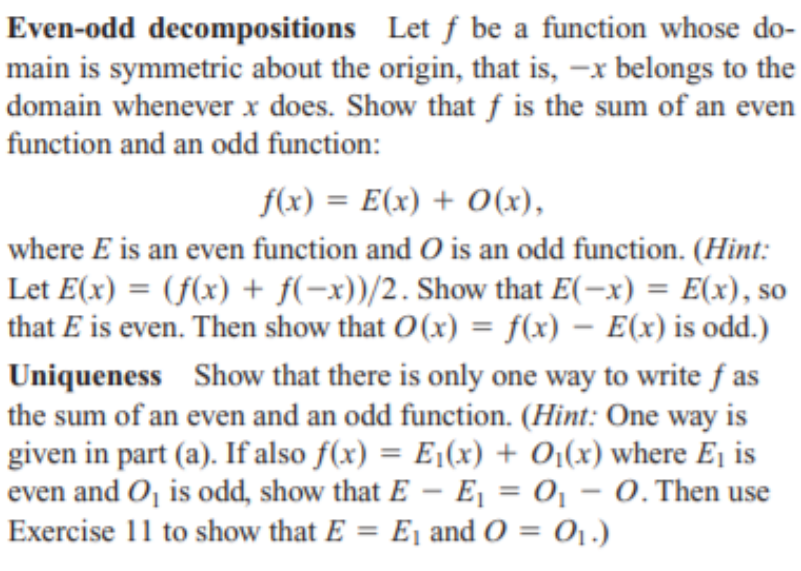
Even$-$odd decompositions Let $f$ be a function whose do$-$

main is symmetric about the origin, that is$,-x$ belongs to the

domain whenever $x$ does. Show that $f$ is the sum of an even

function and an odd function:

$f(x)=E(x)+O(x),$

where $E$ is an even function and $O$ is an odd function. $($Hint:

Let $E(x)=\frac{f(x)+f(-x)}{2}.$ Show that $E(-x)=E(x),$ so

that $E$ is even. Then show that $O(x)=f(x)-E(x)$ is odd.$)$

Uniqueness Show that there is only one way to write $f$ as

the sum of an even and an odd function. $($Hint: One way is

given in part $($a$).$ If also $f(x)=E_1(x)+O_1(x)$ where $E_1$ is

even and $O_1$ is odd, show that $E-E_1=O_1-O.$ Then use

Exercise $11$ to show that $E=E_1$ and $O=O_1.)$