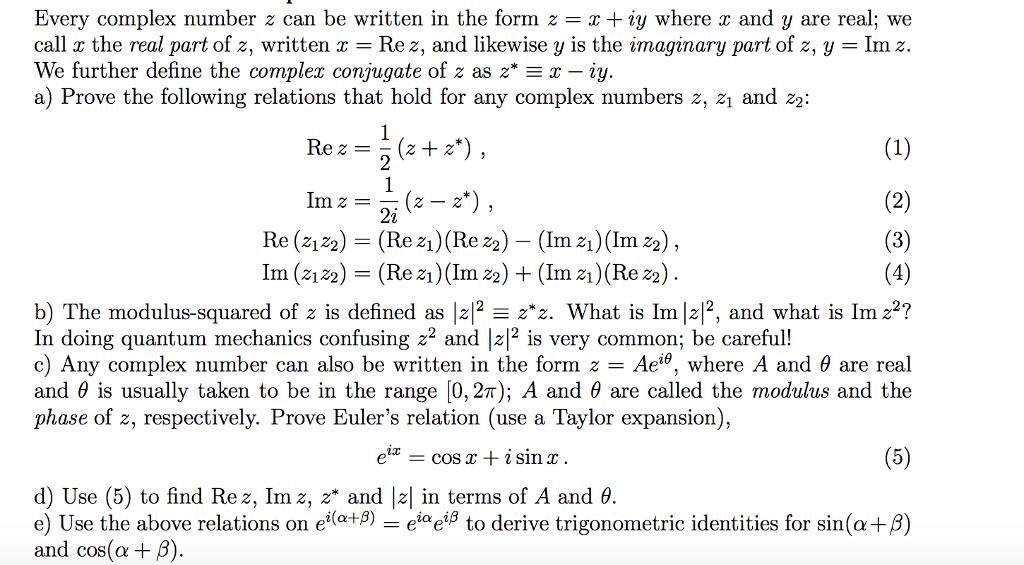
Every complex number z can be written in the form z = x+iy where x and y are real; we call x the real part of z, written x = Rez, and likewise y is the imaginary part of z, y = Im z. We further define the complex conjugate of z as z* = x - iy. a) Prove the following relations that hold for any complex numbers 2, 21 and 22: 1 Rez = (z+z*), 2 1 Im z = (z - 2*), Re (2122) - 2i (Rez1) (Re z2) - (Im 21) (Im z2), (1) (2) (3) (4) Im (2122) (Rez1) (Im z2) + (Im z1) (Re z2). = b) The modulus-squared of z is defined as |2|2 = 2*2. What is Im |2|2, and what is Im z? In doing quantum mechanics confusing 22 and |2|2 is very common; be careful! c) Any complex number can also be written in the form z = Ae, where A and are real and is usually taken to be in the range [0,2); A and 0 are called the modulus and the phase of z, respectively. Prove Euler's relation (use a Taylor expansion), ex cos x + i sin x . d) Use (5) to find Rez, Im z, z* and || in terms of A and 0. e) Use the above relations on ei(a+) and cos(a + B). (5) =eia ei to derive trigonometric identities for sin(a+)