Example 2.10 is in below (even it says 2.8 , it is 2.10)

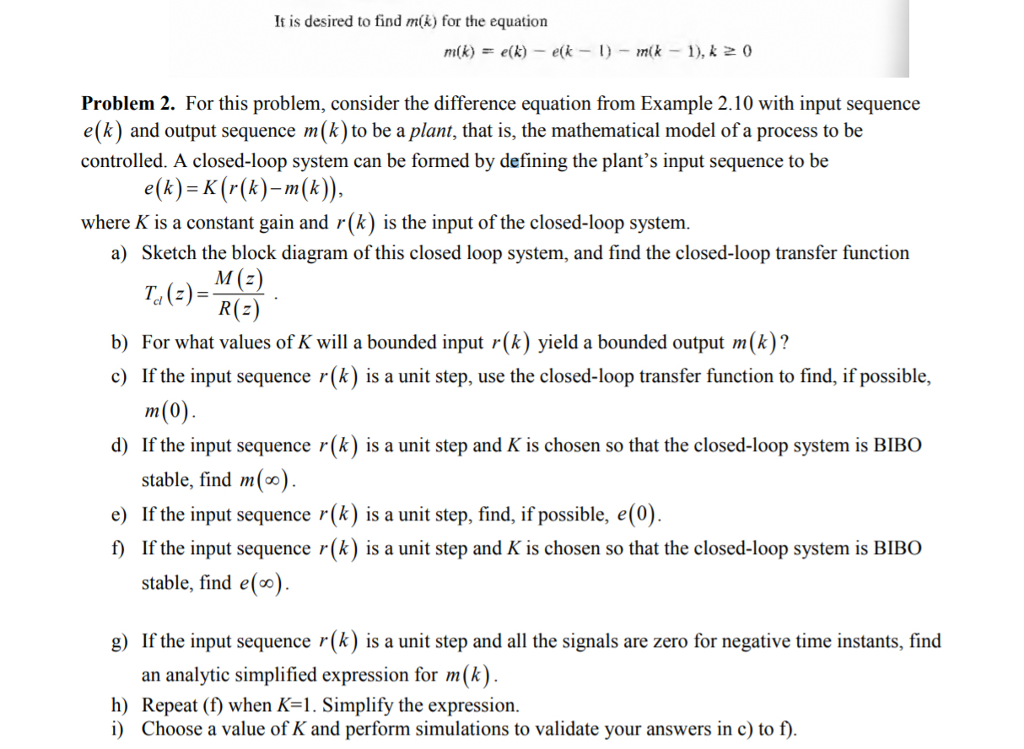
It is desired to find m(k) for the equation Problem 2. For this problem, consider the difference equation from Example 2.10 with input sequence e(k) and output sequence m(k)to be a plant, that is, the mathematical model of a process to be controlled. A closed-loop system can be formed by defining the plant's input sequence to be where K is a constant gain and r(k) is the input of the closed-loop system. a) Sketch the block diagram of this closed loop system, and find the closed-loop transfer function R(2) b) For what values of K will a bounded input r(k) yield a bounded output m(k)? c) If the input sequence r(k) is a unit step, use the closed-loop transfer function to find, if possible, m(0) d) If the input sequence r(k) is a unit step and K is chosen so that the closed-loop system is BIBO stable, find m() If the input sequence r(k) is a unit step, find, if possible, e(0) If the input sequence r(k) is a unit step and K is chosen so that the closed-loop system is BIBO stable, find e(o) e) f) g) If the input sequence r(k) is a unit step and all the signals are zero for negative time instants, find an analytic simplified expression for m(k) h) Repeat (f) when K-1. Simplify the expression i) Choose a value of K and perform simulations to validate your answers in c) to f) Example 2.8 It is desired to find m(k) for the equation m(k) - e(k) e(k1) -m(k 1). k0 where 1.k even e(k) -10.k odd and both e(-1) and m(-1) are zero. Then m(k) can be determined by solving th 0, then for K-1, k-2, and so on. Thus difference equation first for k m(0) = e(0) _ e(-1)-m(-1) = 1-0-0 = 1 m(1)-e(1) e(0(0) 02 m(2)e(2)e)m(1)1-0+2 3 m(3) e(3) e(2) m(2)0-1-3 -4 m(4)-e(4)-e(3)-m(3) = 1-0 + 4 = 5 Note the sequential nature of the solution process in Example 2.8. Using this approach, we can find m(k) for any value of k. This technique is not practical. however, for large values of k, except when implemented on a digital computer. For the example above, a program segment, in MATLAB, which solves the equation for 0 s k s 20 is mkminus1 0; ekminus1 0; for k-0:20 mk = ek-ekmnus1-mkminus1; k.mk] mkminus1 mk; ekminus1- ek; ek 1ek end In the coding, mkminusl represents m(k - 1), mk represents m(k), ekminus represents e(k 1), and ek represents e(k). The first three MATLAB statemenis initialize the sequential process, which begins with the fourth statement and extein to the last statement. A loop exists between the fourth and ninth st first time through the loop mkm(0), the second time through mk -m(l atements and th nd so on Problem 1 a) Run the Matlab code provided in Example 2.10 b) Modify the code to plot the output sequence m(k)'. c) Modify the code to plot the output sequence m(k) when the input sequence is a unit step d) Find the transfer function Is the system with a bounded input sequence e(k) and output E(E) sequence m(k), bounded-input, bounded-output stable? Explain Sample plot of a discrete time sequence or signal: kvec-0:20; %sample time vector mkve c=2 * kvec-20; %sample discrete time vector stem (kvec,mkvec) %Plot the discrete signal of the same dimension as kvec It is desired to find m(k) for the equation Problem 2. For this problem, consider the difference equation from Example 2.10 with input sequence e(k) and output sequence m(k)to be a plant, that is, the mathematical model of a process to be controlled. A closed-loop system can be formed by defining the plant's input sequence to be where K is a constant gain and r(k) is the input of the closed-loop system. a) Sketch the block diagram of this closed loop system, and find the closed-loop transfer function R(2) b) For what values of K will a bounded input r(k) yield a bounded output m(k)? c) If the input sequence r(k) is a unit step, use the closed-loop transfer function to find, if possible, m(0) d) If the input sequence r(k) is a unit step and K is chosen so that the closed-loop system is BIBO stable, find m() If the input sequence r(k) is a unit step, find, if possible, e(0) If the input sequence r(k) is a unit step and K is chosen so that the closed-loop system is BIBO stable, find e(o) e) f) g) If the input sequence r(k) is a unit step and all the signals are zero for negative time instants, find an analytic simplified expression for m(k) h) Repeat (f) when K-1. Simplify the expression i) Choose a value of K and perform simulations to validate your answers in c) to f) Example 2.8 It is desired to find m(k) for the equation m(k) - e(k) e(k1) -m(k 1). k0 where 1.k even e(k) -10.k odd and both e(-1) and m(-1) are zero. Then m(k) can be determined by solving th 0, then for K-1, k-2, and so on. Thus difference equation first for k m(0) = e(0) _ e(-1)-m(-1) = 1-0-0 = 1 m(1)-e(1) e(0(0) 02 m(2)e(2)e)m(1)1-0+2 3 m(3) e(3) e(2) m(2)0-1-3 -4 m(4)-e(4)-e(3)-m(3) = 1-0 + 4 = 5 Note the sequential nature of the solution process in Example 2.8. Using this approach, we can find m(k) for any value of k. This technique is not practical. however, for large values of k, except when implemented on a digital computer. For the example above, a program segment, in MATLAB, which solves the equation for 0 s k s 20 is mkminus1 0; ekminus1 0; for k-0:20 mk = ek-ekmnus1-mkminus1; k.mk] mkminus1 mk; ekminus1- ek; ek 1ek end In the coding, mkminusl represents m(k - 1), mk represents m(k), ekminus represents e(k 1), and ek represents e(k). The first three MATLAB statemenis initialize the sequential process, which begins with the fourth statement and extein to the last statement. A loop exists between the fourth and ninth st first time through the loop mkm(0), the second time through mk -m(l atements and th nd so on Problem 1 a) Run the Matlab code provided in Example 2.10 b) Modify the code to plot the output sequence m(k)'. c) Modify the code to plot the output sequence m(k) when the input sequence is a unit step d) Find the transfer function Is the system with a bounded input sequence e(k) and output E(E) sequence m(k), bounded-input, bounded-output stable? Explain Sample plot of a discrete time sequence or signal: kvec-0:20; %sample time vector mkve c=2 * kvec-20; %sample discrete time vector stem (kvec,mkvec) %Plot the discrete signal of the same dimension as kvec