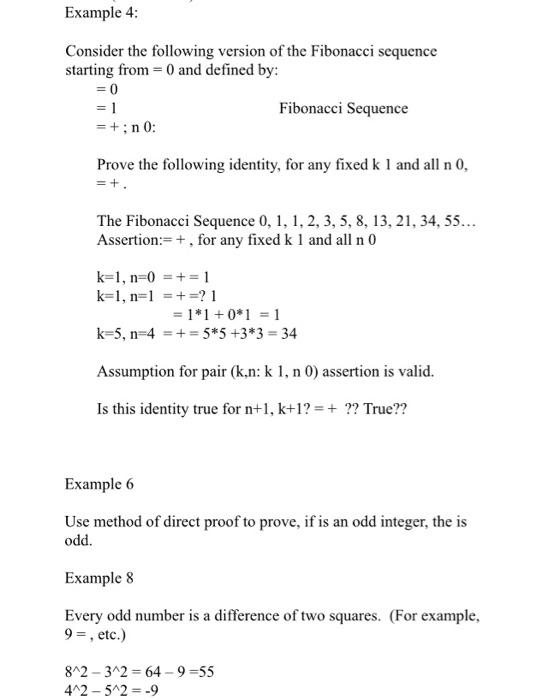
Example 4: Consider the following version of the Fibonacci sequence starting from = 0 and defined by: = 0 = 1 Fibonacci Sequence = +,n0 Prove the following identity, for any fixed k I and all n 0, =+. The Fibonacci Sequence 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55... Assertion:=+, for any fixed k 1 and all n 0 k=1, n=0 = + = 1 k=1, n=1 = + =?1 = 1*1 +0*1 = 1 k=5, n=4 = + = 5*5 +3*3 = 34 Assumption for pair (k.n: k1, n ) assertion is valid. Is this identity true for n+1, k+1? = + ?? True?? Example 6 Use method of direct proof to prove, if is an odd integer, the is odd. Example 8 Every odd number is a difference of two squares. (For example, 9 =, etc.) 8^2-3^2 = 64-9=55 4^2-5^2 = -9 starting from = 0 and defined by: = 0 = 1 Fibonacci Sequence = +:n 0: Prove the following identity, for any fixed k 1 and all n 0. = +. The Fibonacci Sequence 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55... Assertion:= +, for any fixed k 1 and all n 0 k-1, n=0 =+= 1 k=1, n=1 =+=?1 = 1*1 + 0*1 = 1 k=5, n-4 =+= 5*5 +3*3 = 34 Assumption for pair (k.n: kl. n ) assertion is valid. Is this identity true for n+1, k+1? =+ ?? True?? Example 6 Use method of direct proof to prove, if is an odd integer, the is odd. Example 8 Every odd number is a difference of two squares. (For example, 9=, etc.) 812-342-64-9-55 4^2-512 = -9 164-12 Given an odd number x can I find a, b such that x = a^2-b 2 Wrong Q: is the difference of square of any two integers, an odd value? Example 9 Prove by induction that is divisible by 3, for all integers n 1. Example 4: Consider the following version of the Fibonacci sequence starting from = 0 and defined by: = 0 = 1 Fibonacci Sequence = +,n0 Prove the following identity, for any fixed k I and all n 0, =+. The Fibonacci Sequence 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55... Assertion:=+, for any fixed k 1 and all n 0 k=1, n=0 = + = 1 k=1, n=1 = + =?1 = 1*1 +0*1 = 1 k=5, n=4 = + = 5*5 +3*3 = 34 Assumption for pair (k.n: k1, n ) assertion is valid. Is this identity true for n+1, k+1? = + ?? True?? Example 6 Use method of direct proof to prove, if is an odd integer, the is odd. Example 8 Every odd number is a difference of two squares. (For example, 9 =, etc.) 8^2-3^2 = 64-9=55 4^2-5^2 = -9 starting from = 0 and defined by: = 0 = 1 Fibonacci Sequence = +:n 0: Prove the following identity, for any fixed k 1 and all n 0. = +. The Fibonacci Sequence 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55... Assertion:= +, for any fixed k 1 and all n 0 k-1, n=0 =+= 1 k=1, n=1 =+=?1 = 1*1 + 0*1 = 1 k=5, n-4 =+= 5*5 +3*3 = 34 Assumption for pair (k.n: kl. n ) assertion is valid. Is this identity true for n+1, k+1? =+ ?? True?? Example 6 Use method of direct proof to prove, if is an odd integer, the is odd. Example 8 Every odd number is a difference of two squares. (For example, 9=, etc.) 812-342-64-9-55 4^2-512 = -9 164-12 Given an odd number x can I find a, b such that x = a^2-b 2 Wrong Q: is the difference of square of any two integers, an odd value? Example 9 Prove by induction that is divisible by 3, for all integers n 1