Answered step by step
Verified Expert Solution
Question
1 Approved Answer
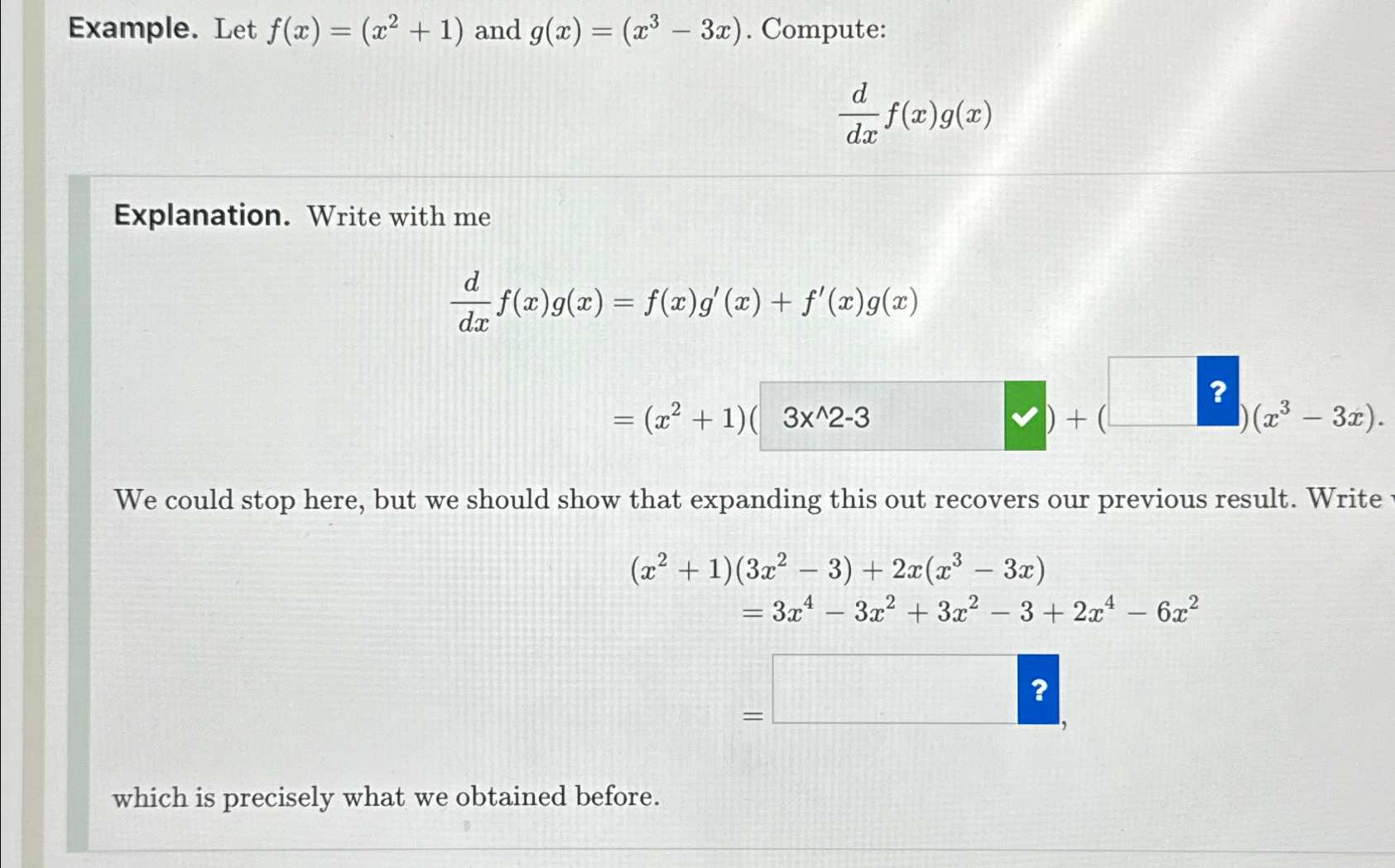
Example. Let f(x)=(x^(2)+1) and g(x)=(x^(3)-3x) . Compute: (d)/(dx)f(x)g(x) Explanation. Write with me (d)/(dx)f(x)g(x)=f(x)g^(')(x)+f^(')(x)g(x) =(x^(2)+1) We could stop here, but we should show that
Example. Let
f(x)=(x^(2)+1)and
g(x)=(x^(3)-3x). Compute:\
(d)/(dx)f(x)g(x)\ Explanation. Write with me\
(d)/(dx)f(x)g(x)=f(x)g^(')(x)+f^(')(x)g(x)\ =(x^(2)+1)\ We could stop here, but we should show that expanding this out recovers our previous result. Write\
(x^(2)+1)(3x^(2)-3)+2x(x^(3)-3x)\ =3x^(4)-3x^(2)+3x^(2)-3+2x^(4)-6x^(2)\ which is precisely what we obtained before.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


