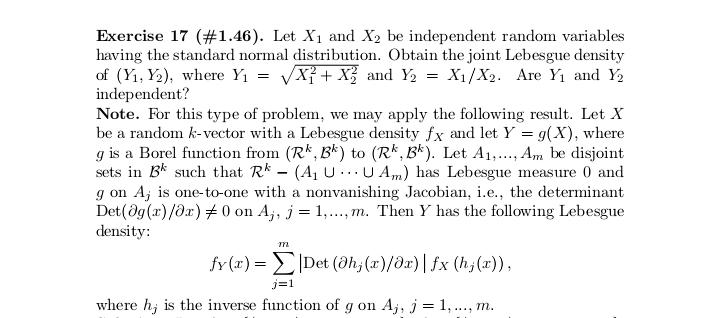






Exercise 17 (#1.46). Let X1 and X2 be independent random variables having the standard normal distribution. Obtain the joint Lebesgue density of (Yi, Y2), where Y1 = VX7 + X, and Y2 = X1/X2. Are Yi and Y2 independent? Note. For this type of problem, we may apply the following result. Let X be a random k-vector with a Lebesgue density fx and let Y = g(X), where g is a Borel function from (R*, B*) to (R*, B*). Let A1. . ... Am be disjoint sets in B* such that R* - (Aj U . .. U Am,) has Lebesgue measure () and g on A, is one-to-one with a nonvanishing Jacobian, i.e., the determinant Det(Og(x) /Or) 0 on A;, j = 1. ...,m. Then Y has the following Lebesgue density: fy(x) = >|Det (Oh, (x)/Or) | fx (h;(x)) where h, is the inverse function of g on Aj, j = 1, .... m.Problem 7.23. The one-year LIBOR rate is 3% and the forward rate for the one- to two-year period is 3.2% The three-year swap rate for a swap with annual payments is 3.2%. What is the LIBOR forward rate for the 2 to 3 year period if OIS discounting is used and the OIS zero rates for one, two, and three year maturities are 2.5%, 2.7%, and 2.9%, respectively. What is the value of a three-year swap where 4% is received and LIBOR is paid on a principal of $100 million. All rates are annually compounded.Problem 7.13. After it hedges its foreign exchange risk using forward contracts, is the financial institution's average spread in Figure 7.11 likely to be greater than or less than 20 basis points? Explain your answer.11:58 P -O ... OB/s O Mil all 1 17% 42. Prices for 6-month 60-strike European up-and-out call options on a stock S are available. Below is a table of option prices with respect to various /. the level of the barrier. Here, S(0) = 50. Price of up-and-out call 60 70 0.1294 80 0.7583 90 1.6616 4.0861 Consider a special 6-month 60-strike European "knock-in, partial knock-out" call option that knocks in at #1 = 70, and "partially" knocks out at #2 - 80. The strike price of the option is 60. The following table summarizes the payoff at the exercise date: HI Hit Hi Not Hit He Not Hit He Hit 0 2 x max [ $(0.5) - 60, 0] max [ S(0.5) -60, 0] Calculate the price of the option. (A) 06289 (B) 1.3872 (C) 21455 (D) 45856 (E) I cannot be determined from the information given above. 43. DELETED IFM-01-18 Page 54 of 105 44. Consider the following three-period binomial tree model for a stock that pays dividends continuously at a rate proportional to its price. The length of each period is 1 year, the continuously compounded risk-free interest rate is 10%, and the continuous dividend yield on the stock is 6.5%. 585.9375 468.75 375 328.125 300 262.5 210 183.75 147 102.9 Calculate the price of a 3-year at-the-money American put option on the stock. (A) 15.86 (B) 27.40 (9) 32.60 O O20) Since the development of the CAPM model, it is not uncommon that practitioners use market capitalization, book-to-market ratio and past returns to form portfolios that have a positive alpha. Thus, the market portfolio may not be efficient, and therefore a stock's beta is not an adequate measure of systematic risk. Determine which of the following is NOT a reason why a market portfolio may not be efficient. (A) Alternative Risk Preferences (B) Non-Tradable Wealth (9) Proxy Error (D) Behavioral Biases (E) No portfolios are efficientProblem 7.14. "Companies with high credit risks are the ones that cannot access fixed-rate markets directly. They are the companies that are most likely to be paying fixed and receiving floating in an interest rate swap. " Assume that this statement is true. Do you think it increases or decreases the risk of a financial institution's swap portfolio? Assume that companies are most likely to default when interest rates are high.5. The PS index has the following characteristics: One share of the PS index currently sells for 1,000. The PS index does not pay dividends. Sam wants to lock in the ability to buy this index in one year for a price of 1,025. He can do this by buying or selling European put and call options with a strike price of 1,025. The annual effective risk-free interest rate is 5%. Determine which of the following gives the hedging strategy that will achieve Sam's objective and also gives the cost today of establishing this position




















