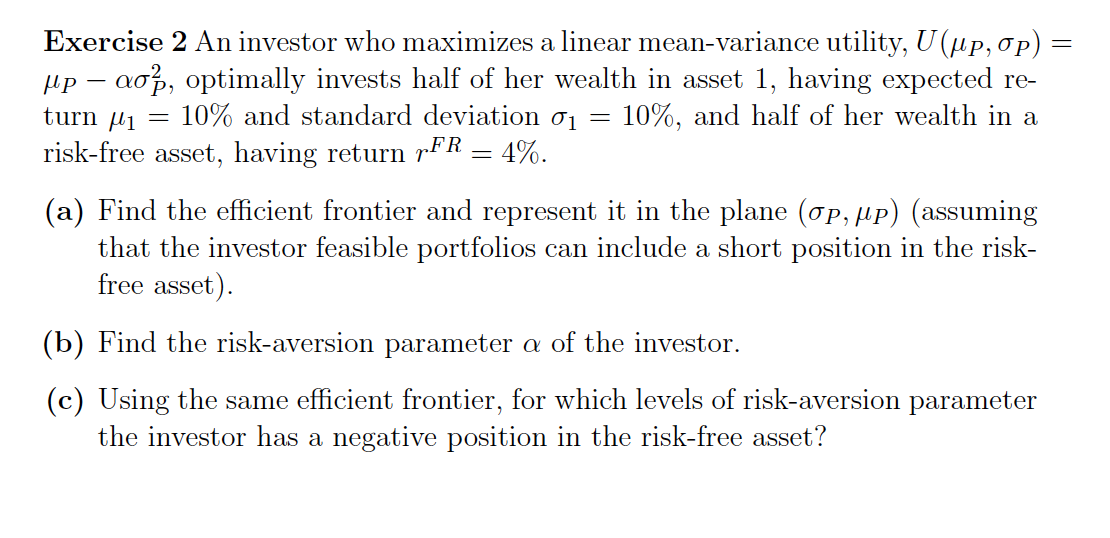
Exercise $2$ An investor who maximizes a linear mean$-$variance utility, $U({}_P,{}_P)=$

${}_P-{}_P^2,$ optimally invests half of her wealth in asset $1,$ having expected re$-$

turn ${}_1=10\%$ and standard deviation ${}_1=10\%,$ and half of her wealth in a

risk$-$free asset, having return $r^{FR}=4\%.$

$($a$)$ Find the efficient frontier and represent it in the plane $({}_P,{}_P)($assuming

that the investor feasible portfolios can include a short position in the risk$-$

free asset$).$

$($b$)$ Find the risk$-$aversion parameter $$ of the investor.

$($c$)$ Using the same efficient frontier, for which levels of risk$-$aversion parameter

the investor has a negative position in the risk$-$free asset?