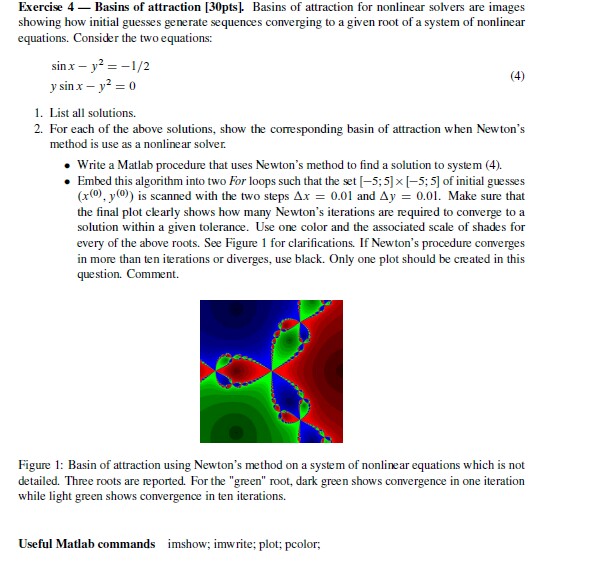
Exercise 4- Basins of attraction [30pts Basins of attraction for nonlinear solvers are images showing how initial guesses generate sequences converging to a given root of a system of nonlinear equations. Consider the two equations: sinx - y2--1/2 y sin xy0 1. List all solutions. 2. For each of the above solutions, show the corresponding basin of attraction when Newton's method is use as a nonline ar solver. . Write a Matlab procedure that uses Newton's method to find a solution to system (4). . Embed this algorithm into two For loops such that the set-5;5]x -5;5 of initial guesses (x(0), y(0) is scanned with the two steps x = 0.01 and y = 0.01. Make sure that the final plot clearly shows how many Newton's iterations are required to converge to a solution within a given tolerance. Use one color and the associated scale of shades for every of the above roots. See Figure 1 for clarifications. If Newton's procedure converges in more than ten iterations or diverges, use black. Only one plot should be created in this question. Comment. Figure 1: Basin of attraction using Newton's method on a system of nonlinear equations which is not detailed. Three roots are reported. For the "green" root, dark green shows convergence in one iteration while light green shows convergence in ten iterations. Useful Matlab commands imshow; imwrite; plot; pcolor; Exercise 4- Basins of attraction [30pts Basins of attraction for nonlinear solvers are images showing how initial guesses generate sequences converging to a given root of a system of nonlinear equations. Consider the two equations: sinx - y2--1/2 y sin xy0 1. List all solutions. 2. For each of the above solutions, show the corresponding basin of attraction when Newton's method is use as a nonline ar solver. . Write a Matlab procedure that uses Newton's method to find a solution to system (4). . Embed this algorithm into two For loops such that the set-5;5]x -5;5 of initial guesses (x(0), y(0) is scanned with the two steps x = 0.01 and y = 0.01. Make sure that the final plot clearly shows how many Newton's iterations are required to converge to a solution within a given tolerance. Use one color and the associated scale of shades for every of the above roots. See Figure 1 for clarifications. If Newton's procedure converges in more than ten iterations or diverges, use black. Only one plot should be created in this question. Comment. Figure 1: Basin of attraction using Newton's method on a system of nonlinear equations which is not detailed. Three roots are reported. For the "green" root, dark green shows convergence in one iteration while light green shows convergence in ten iterations. Useful Matlab commands imshow; imwrite; plot; pcolor