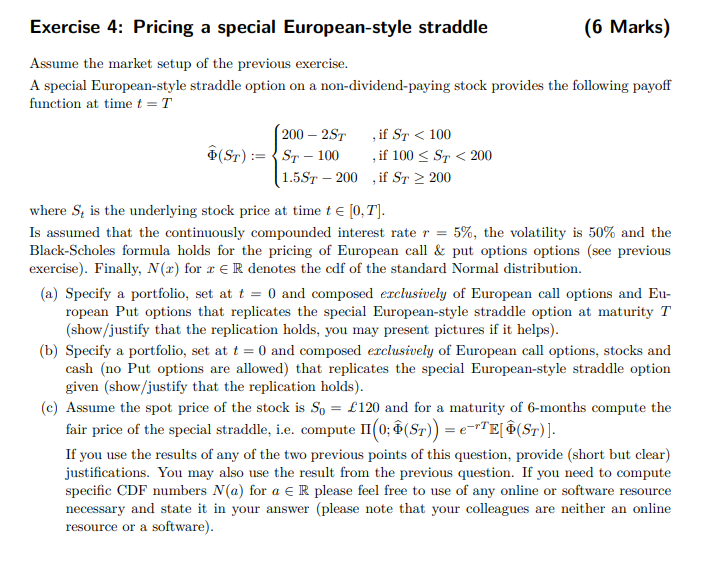
Exercise 4: Pricing a special European-style straddle (6 Marks) Assume the market setup of the previous exercise. A special European-style straddle option on a non-dividend-paying stock provides the following payoff function at time t =T 200 - 25T , if ST 200 where S4 is the underlying stock price at time t (0,T]. Is assumed that the continuously compounded interest rate r = 5%, the volatility is 50% and the Black-Scholes formula holds for the pricing of European call & put options options (see previous exercise). Finally, N() for R denotes the cdf of the standard Normal distribution. (a) Specify a portfolio, set at t = 0 and composed exclusively of European call options and Eu- ropean Put options that replicates the special European-style straddle option at maturity T (show/justify that the replication holds, you may present pictures if it helps). (b) Specify a portfolio, set at t = 0 and composed exclusively of European call options, stocks and cash (no Put options are allowed) that replicates the special European-style straddle option given (show/justify that the replication holds). (e) Assume the spot price of the stock is so = 120 and for a maturity of 6-months compute the fair price of the special straddle, i.e. compute 11(0; (Sr)) = e--TE[ (ST)]. If you use the results of any of the two previous points of this question, provide (short but clear) justifications. You may also use the result from the previous question. If you need to compute specific CDF numbers N(a) for a R please feel free to use of any online or software resource necessary and state it in your answer (please note that your colleagues are neither an online resource or a software). Exercise 4: Pricing a special European-style straddle (6 Marks) Assume the market setup of the previous exercise. A special European-style straddle option on a non-dividend-paying stock provides the following payoff function at time t =T 200 - 25T , if ST 200 where S4 is the underlying stock price at time t (0,T]. Is assumed that the continuously compounded interest rate r = 5%, the volatility is 50% and the Black-Scholes formula holds for the pricing of European call & put options options (see previous exercise). Finally, N() for R denotes the cdf of the standard Normal distribution. (a) Specify a portfolio, set at t = 0 and composed exclusively of European call options and Eu- ropean Put options that replicates the special European-style straddle option at maturity T (show/justify that the replication holds, you may present pictures if it helps). (b) Specify a portfolio, set at t = 0 and composed exclusively of European call options, stocks and cash (no Put options are allowed) that replicates the special European-style straddle option given (show/justify that the replication holds). (e) Assume the spot price of the stock is so = 120 and for a maturity of 6-months compute the fair price of the special straddle, i.e. compute 11(0; (Sr)) = e--TE[ (ST)]. If you use the results of any of the two previous points of this question, provide (short but clear) justifications. You may also use the result from the previous question. If you need to compute specific CDF numbers N(a) for a R please feel free to use of any online or software resource necessary and state it in your answer (please note that your colleagues are neither an online resource or a software)







