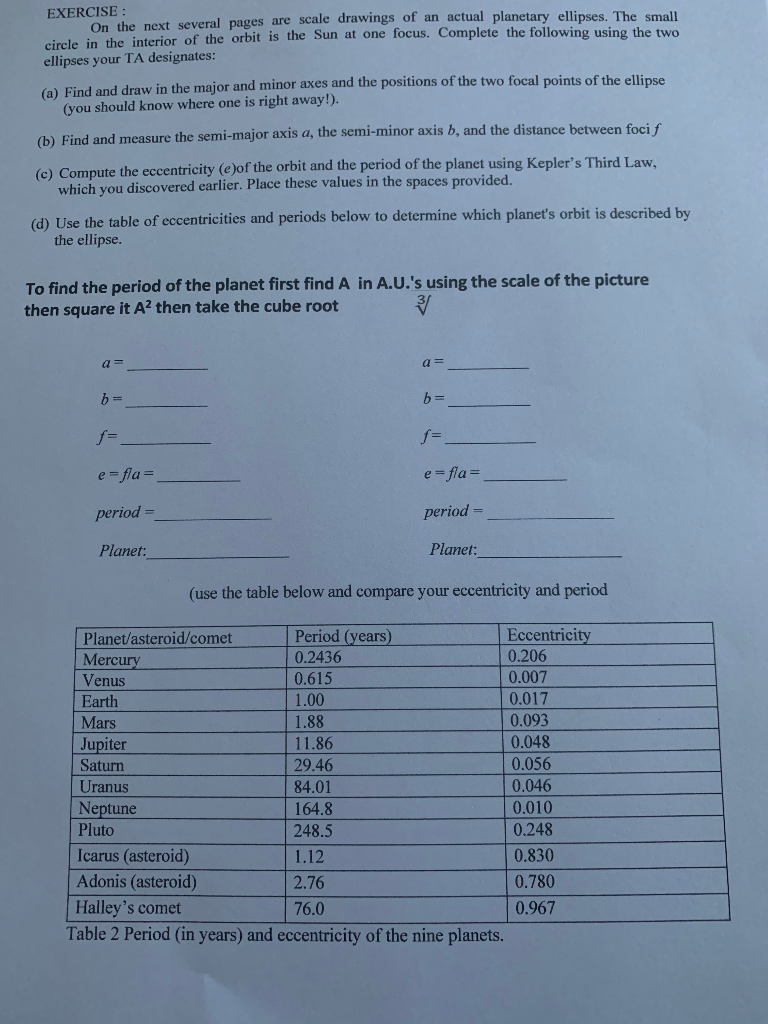

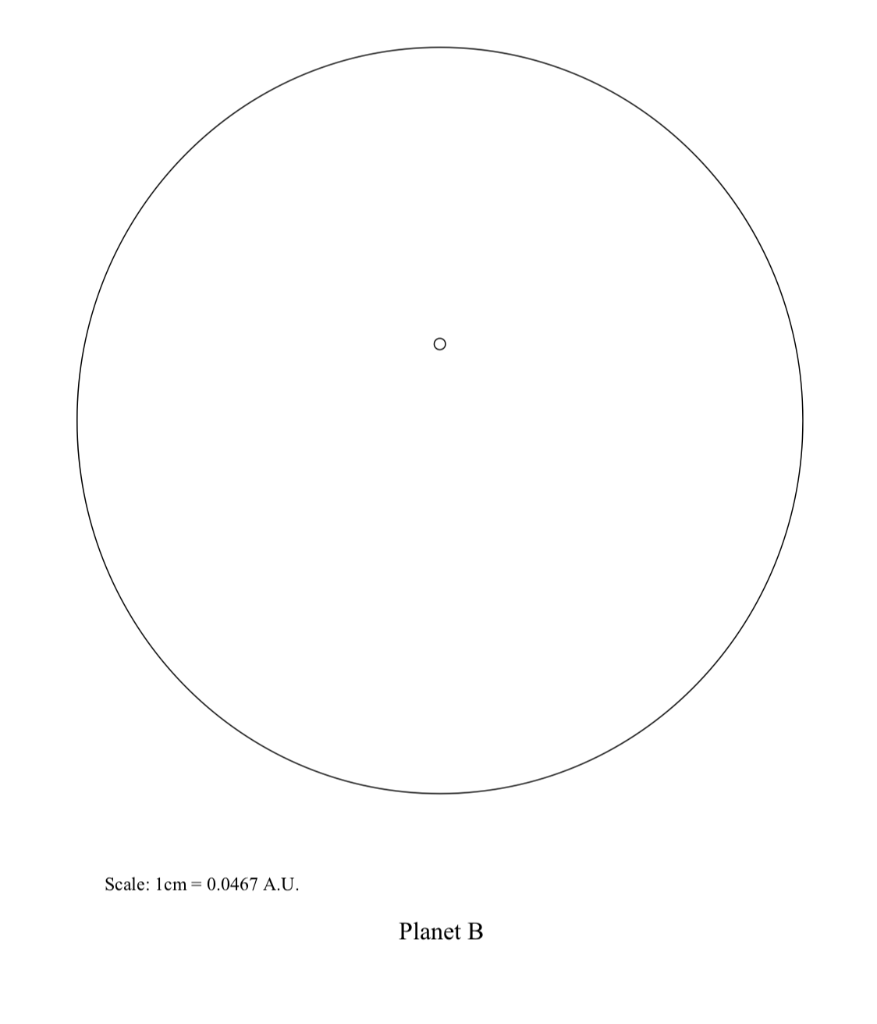
EXERCISE On the next several pages are scale drawings of an actual planetary ellipses. The small circle in the interior of the orbit is the Sun at one focus. Complete the following using the two ellipses your TA designates: (a) Find and draw in the major and minor axes and the positions of the two focal points of the ellipse (you should know where one is right away!). (b) Find and measure the semi-major axis a, the semi-minor axis b, and the distance between focif (c) Compute the eccentricity (e)of the orbit and the period of the planet using Kepler's Third Law, which you discovered earlier. Place these values in the spaces provided. (d) Use the table of eccentricities and periods below to determine which planet's orbit is described by the ellipse. To find the period of the planet first find A in A.U.'s using the scale of the picture then square it A2 then take the cube root a= b= b= f= f= e = fla= e=fla= period period = Planet: Planet: (use the table below and compare your eccentricity and period Planet/asteroid/comet Period (years) Eccentricity Mercury 0.2436 0.206 Venus 0.615 0.007 Earth 1.00 0.017 Mars 1.88 0.093 Jupiter 11.86 0.048 Saturn 29.46 0.056 Uranus 84.01 0.046 Neptune 164.8 0.010 Pluto 248.5 Icarus (asteroid) 1.12 0.830 Adonis (asteroid) 2.76 0.780 Halley's comet 76.0 0.967 Table 2 Period (in years) and eccentricity of the nine planets. 0.248 Note: 1cm=0.186AU Planet A o Inside 3-2 O Scale: 1cm=0.0467 A.U. Planet B EXERCISE On the next several pages are scale drawings of an actual planetary ellipses. The small circle in the interior of the orbit is the Sun at one focus. Complete the following using the two ellipses your TA designates: (a) Find and draw in the major and minor axes and the positions of the two focal points of the ellipse (you should know where one is right away!). (b) Find and measure the semi-major axis a, the semi-minor axis b, and the distance between focif (c) Compute the eccentricity (e)of the orbit and the period of the planet using Kepler's Third Law, which you discovered earlier. Place these values in the spaces provided. (d) Use the table of eccentricities and periods below to determine which planet's orbit is described by the ellipse. To find the period of the planet first find A in A.U.'s using the scale of the picture then square it A2 then take the cube root a= b= b= f= f= e = fla= e=fla= period period = Planet: Planet: (use the table below and compare your eccentricity and period Planet/asteroid/comet Period (years) Eccentricity Mercury 0.2436 0.206 Venus 0.615 0.007 Earth 1.00 0.017 Mars 1.88 0.093 Jupiter 11.86 0.048 Saturn 29.46 0.056 Uranus 84.01 0.046 Neptune 164.8 0.010 Pluto 248.5 Icarus (asteroid) 1.12 0.830 Adonis (asteroid) 2.76 0.780 Halley's comet 76.0 0.967 Table 2 Period (in years) and eccentricity of the nine planets. 0.248 Note: 1cm=0.186AU Planet A o Inside 3-2 O Scale: 1cm=0.0467 A.U. Planet B









