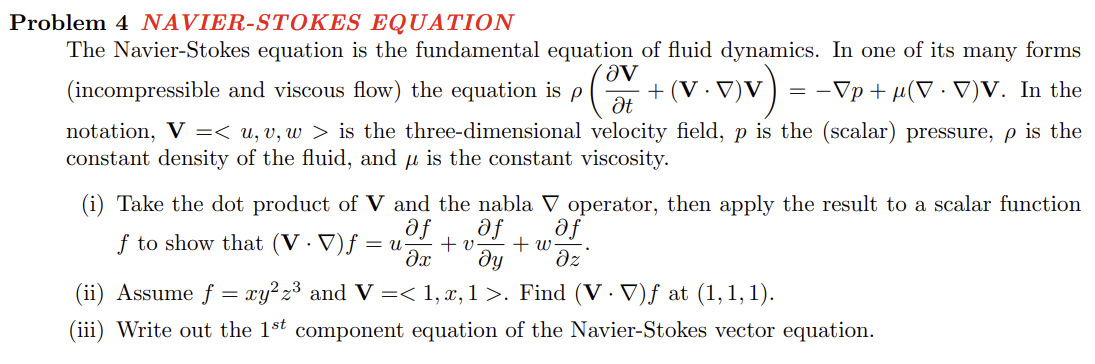
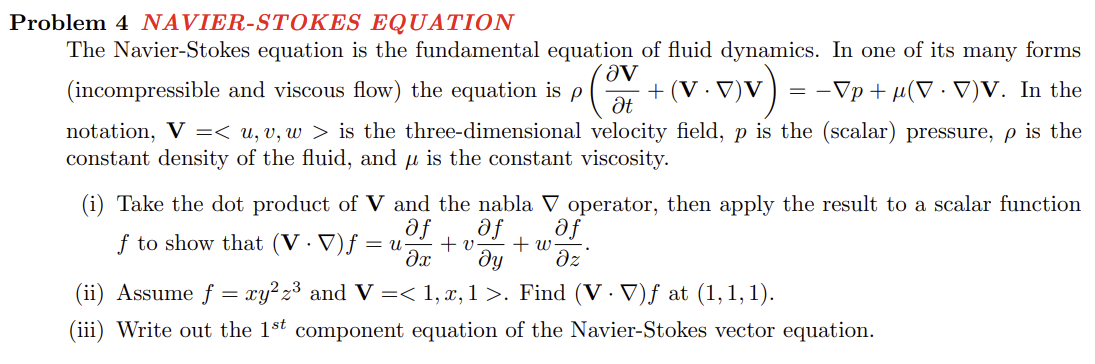
NAVIER-STOKES EQUATION Problem 4 The Navier-Stokes equation is the fundamental equation of fluid dynamics. In one of its many forms (incompressible and viscous flow) the equation is p VP II(S7 . V) V. In the notation, V u, v, w > is the three-dimensional velocity field, p is the (scalar) pressure, p is the constant density of the fluid, and is the constant viscosity. (i) (ii) (iii) Take the dot product of V and the nabla V operator, then apply the result to a scalar function Of Of Of f to show that (V V) f u -k t, + u, Oz Assume f xy2z3 and V 1, x, 1 >. Find (V V) f at (1, 1, 1). Write out the ISt component equation of the Navier-Stokes vector equation.