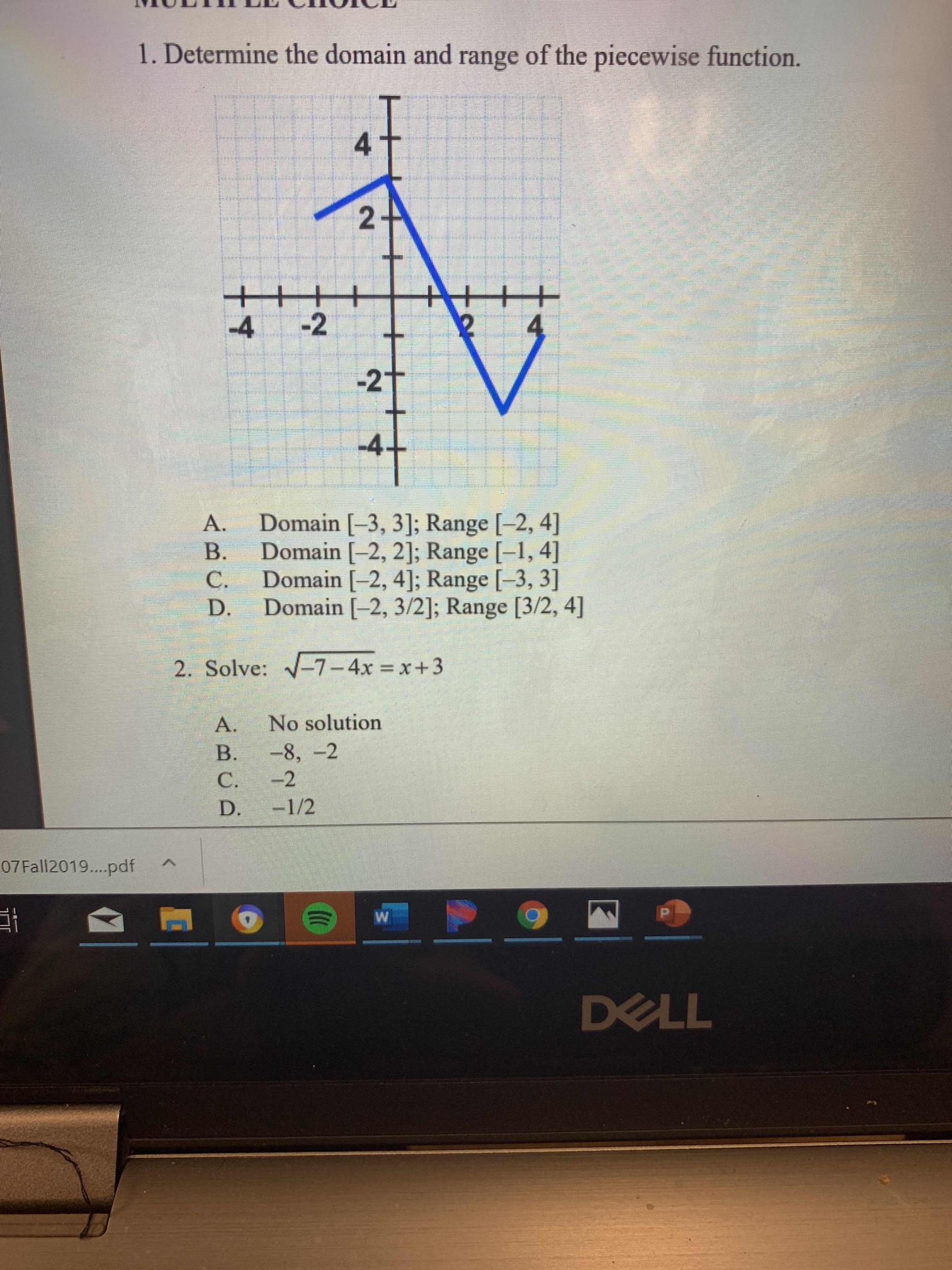
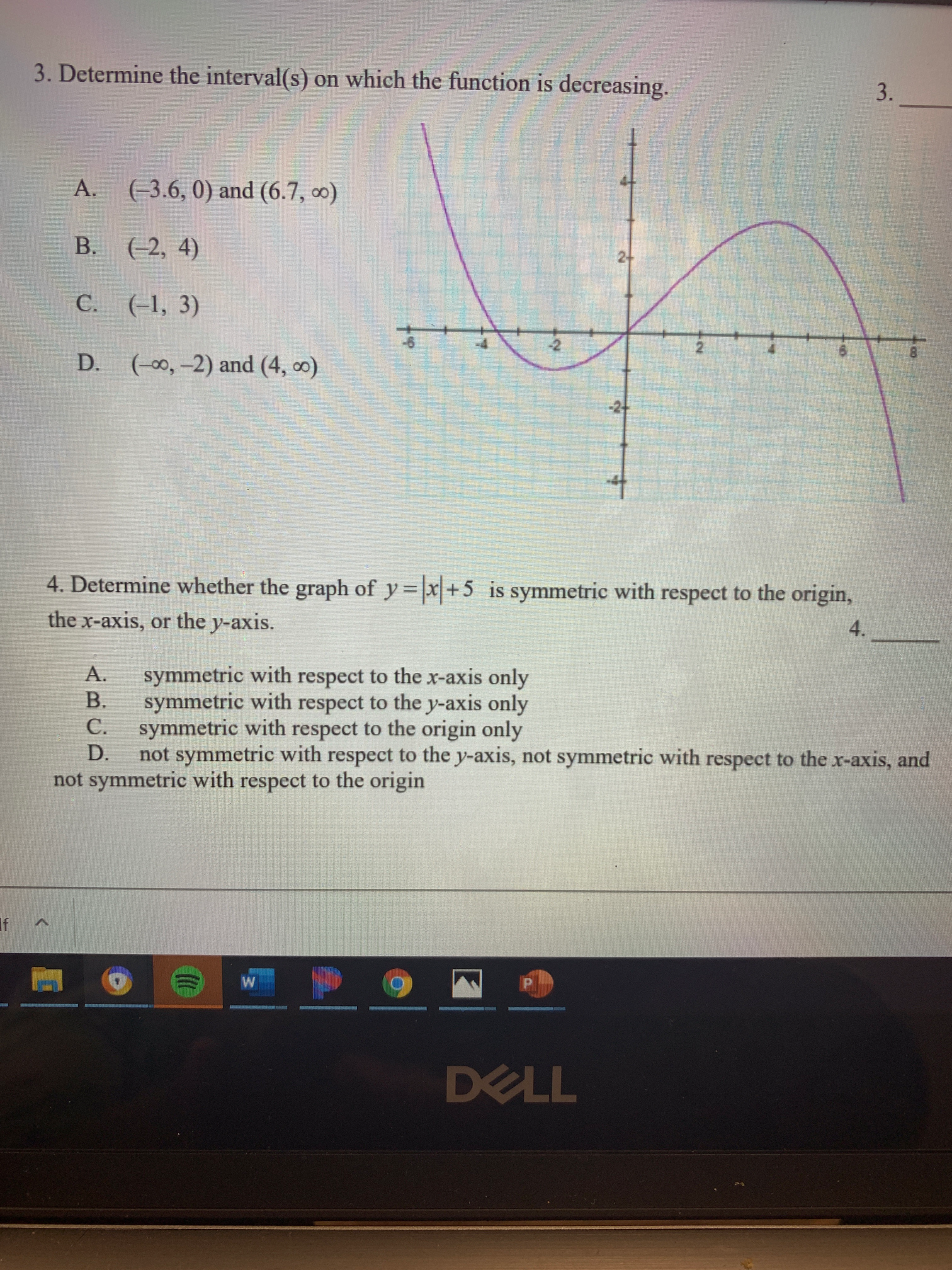
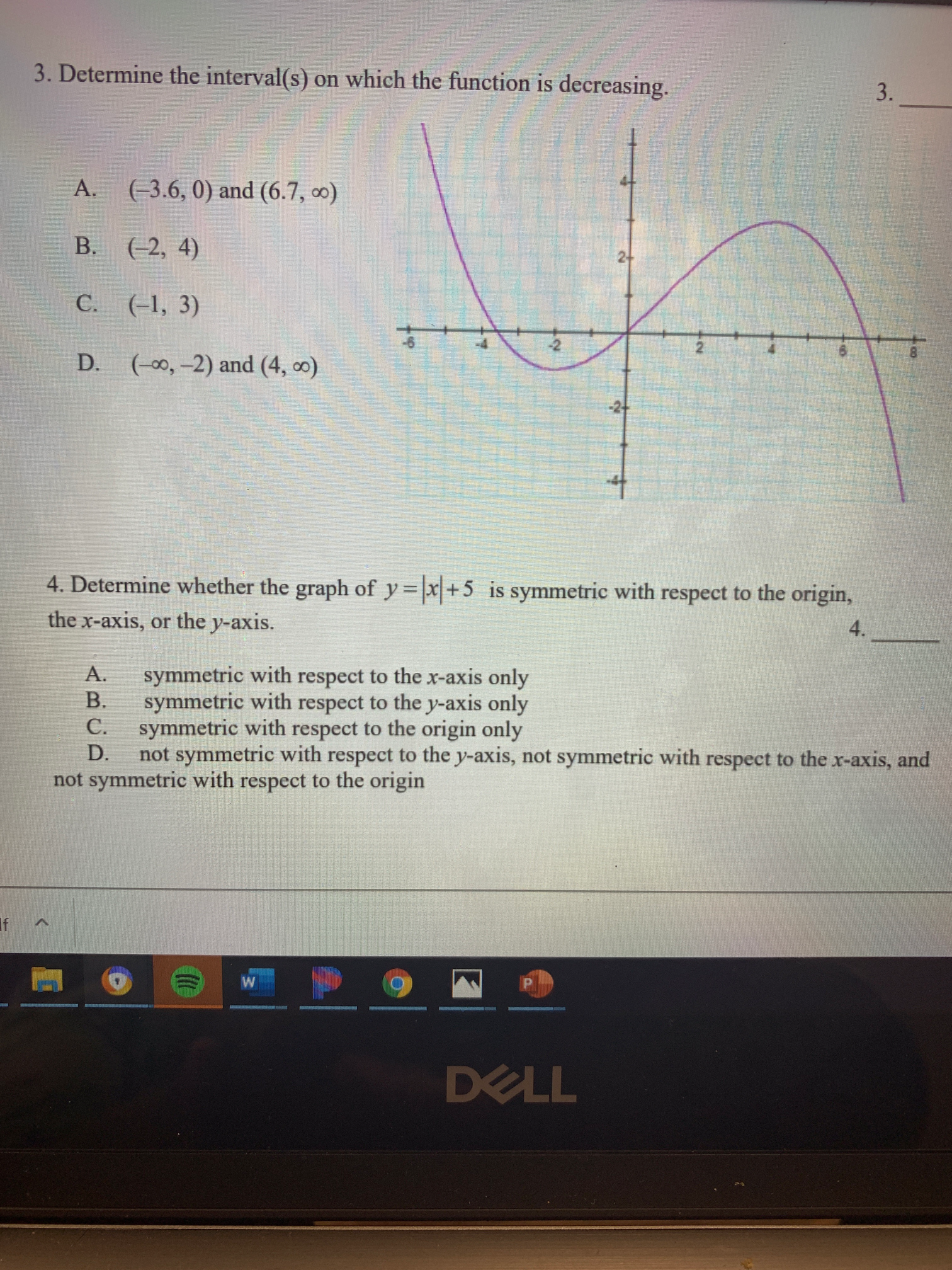
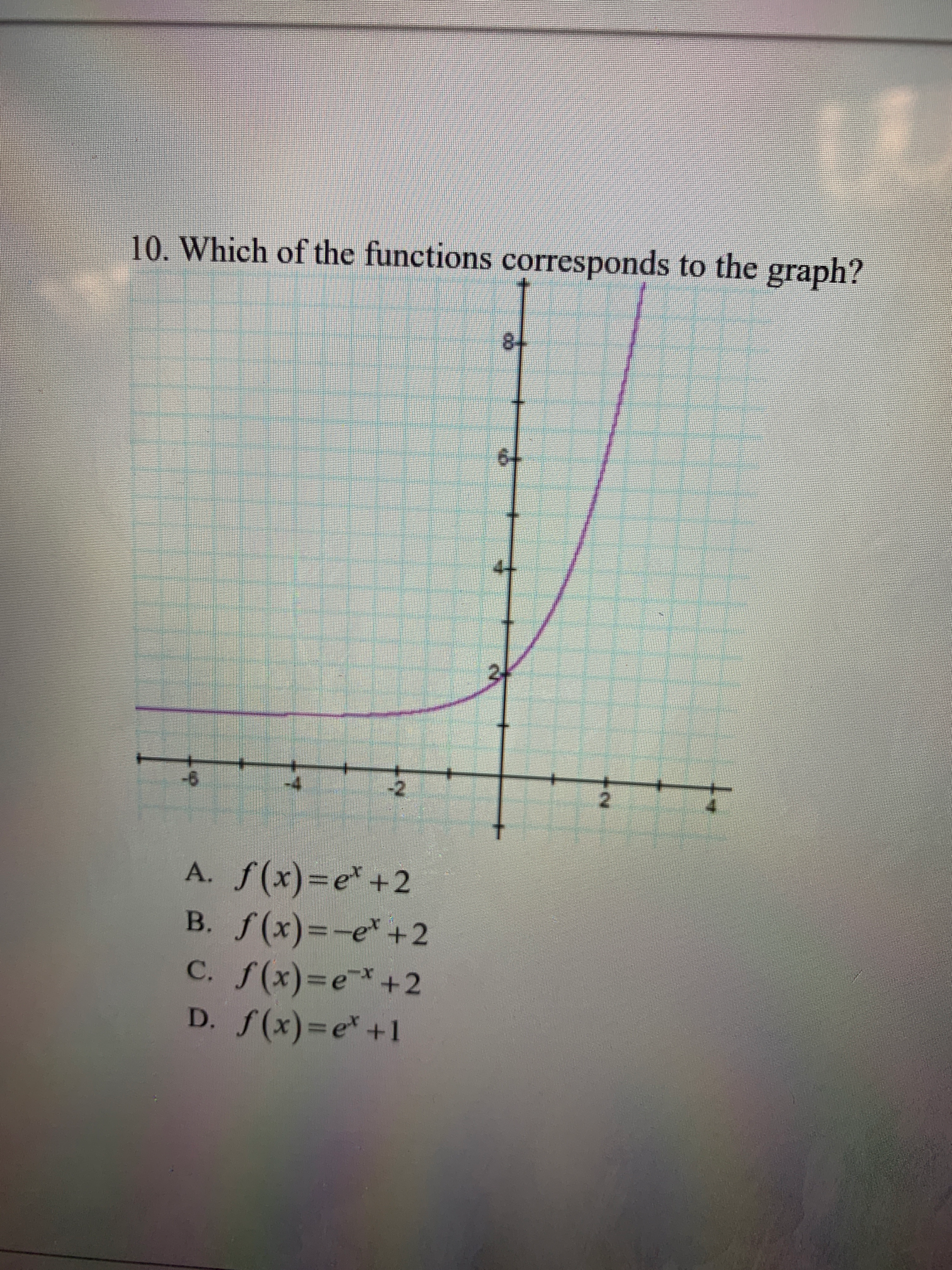
\f5. Solve, and express the answer in interval notation: | 7 - 9x | 2 20. A. (-00, -13/9] ~ [3, 00) B. (-00, 3] C. [-13/9, 3] D. [-13/9, 00) College Algebra MATH 107 ....pdf A W P DELLPage 3 of 11 College Algebra MATH 107 Fall, 2019, V1.15 7. Write a slope-intercept equation for a line parallel to the line x - 8y = 16 which passes through the point (24, -1). A. y = - -x-2 B. y = x-4 C. y=-x-1 D. y=-2x+47 8 Which of the following best describes the graph? 2019....pdf w P DELL5. Solve, and express the answer in interval notation: | 7 - 9x | 2 20. A. (-00, -13/9] ~ [3, 00) B. (-00, 3] C. [-13/9, 3] D. [-13/9, 00) College Algebra MATH 107 ....pdf A W P DELLCollege Algebra MATH 107 9. Express as an equivalent expression: 8 log (x - 3) + log 1 - logy A. log(x -3) log(y) B. log 8x - 24 C. log (x-3) y D. log (8x-23- y) 10. Which of the functions corresponds to the graph?3. Determine the interval(s) on which the function is decreasing. A. (-3.6, 0) and (6.7, co) B. (-2, 4) C. (-1, 3) D. (-0o, -2) and (4, 00) 2 4. Determine whether the graph of y = \\x|+5 is symmetric with respect to the origin, the x-axis, or the y-axis. A. symmetric with respect to the x-axis only B. symmetric with respect to the y-axis only C. symmetric with respect to the origin only D. not symmetric with respect to the y-axis, not symmetric with respect to the x-axis, and not symmetric with respect to the origin w 19 P DELL3. Determine the interval(s) on which the function is decreasing. A. (-3.6, 0) and (6.7, co) B. (-2, 4) C. (-1, 3) D. (-0o, -2) and (4, 00) 2 4. Determine whether the graph of y = \\x|+5 is symmetric with respect to the origin, the x-axis, or the y-axis. A. symmetric with respect to the x-axis only B. symmetric with respect to the y-axis only C. symmetric with respect to the origin only D. not symmetric with respect to the y-axis, not symmetric with respect to the x-axis, and not symmetric with respect to the origin w 19 P DELL10. Which of the functions corresponds to the graph? A. f(x) = ex+2 B. f(x) =-ex+2 c. f ( x ) = ex + 2 D. f(x) = ex+1