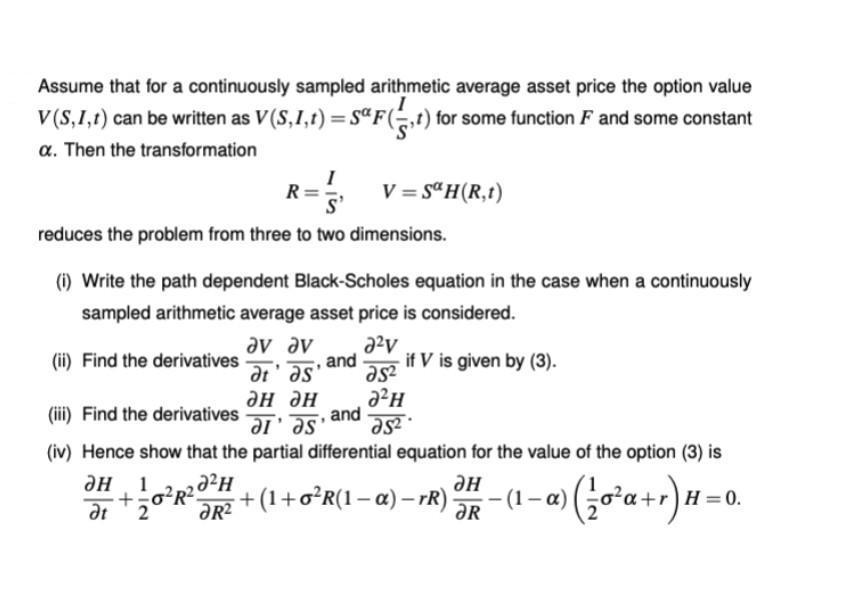
Assume that for a continuously sampled arithmetic average asset price the option value V(S,1,1) can be written as V(S,1,1)=SF(t) for some function F and some constant a. Then the transformation R= = reduces the problem from three to two dimensions. I 1 +0R5 2 t V = SaH(R,t) (i) Write the path dependent Black-Scholes equation in the case when a continuously sampled arithmetic average asset price is considered. 2v (ii) Find the derivatives av av and if V is given by (3). dat as' 25 2 and d'as' 25 (iii) Find the derivatives (iv) Hence show that the partial differential equation for the value of the option (3) is (1-a) ( oa+r) H=0. - R H H - + (1 + oR(1 a) rR) JR -