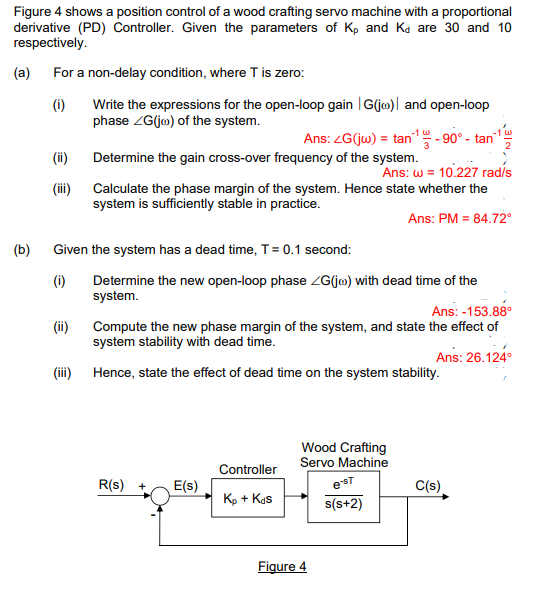
Figure 4 shows a position control of a wood crafting servo machine with a proportional derivative (PD) Controller. Given the parameters of Kp and Kd are 30 and 10 respectively. (a) For a non-delay condition, where T is zero: (i) Write the expressions for the open-loop gain | G(jo) and open-loop phase G(jo) of the system. (ii) Ans: G(jw) = tan-90 - tan Determine the gain cross-over frequency of the system. Ans: w = 10.227 rad/s (iii) Calculate the phase margin of the system. Hence state whether the system is sufficiently stable in practice. Ans: PM = 84.72 (b) Given the system has a dead time, T = 0.1 second: (i) (ii) (iii) Determine the new open-loop phase ZG(j) with dead time of the system. Ans: -153.88 Compute the new phase margin of the system, and state the effect of system stability with dead time. Hence, state the effect of dead time on the system stability. Ans: 26.124 Wood Crafting Servo Machine Controller R(s) + E(s) e-ST C(s) Kp + Kds s(s+2) Figure 4